Abstract
The paper concentrates on the study of reflection and transmission characteristics of waves at the interface of a thermoelastic-diffusive solid half-space underlying an inviscid liquid. The analytic expressions for amplitude ratios, reflection and transmission coefficients, in terms of incident angles and material parameters have been obtained for quasi-longitudinal (
qP
) and quasi-transverse (qSV
) wave incidence. The normal and grazing incidence cases have also been derived and discussed. The total reflection phenomenon has also been discussed. The energy law has been shown to be obeyed by the incidence, reflected and transmitted waves by deriving energy equation and its simulation. The numerical computations of reflection and transmission coefficients have been carried out for copper material half-space in contact with water at the interface by using MATLAB software. The computer simulated results have been presented graphically in order to bring out clear comparison of various situations.Keywords
Thermoelastic Diffusion, Inviscid fluid, Reflection, Transmission, Energy Ratios, Critical angle.
Study of reflection and transmission of plane waves
at thermoelastic-diffusive solid/liquid interface
1 INTRODUCTION
Diffusion is defined as a movement of the particles from a region of high concentration to the low concentration. Thermal diffusion utilizes the transfer of heat across a thin liquid or gas to accomplish isotope separation. Today, the study of thermal diffusion phenomenon got a great deal of interest due to its wide ranging applications in geophysics and industrial applications. The concentration obeys the famous Fick’s law, which does not take into consideration the mutual interaction between the solvent and the solute or the effect of temperature on this interaction.
J.N. Sharma a R. Kaur b
Department of Mathematics, National Institute of Technology
Hamirpur-177005, India a
jnsnith@gmail.com b
kaur.rajbir22@gmail.com
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
However, there is a certain degree of coupling with temperature and temperature gradients as temperature speeds up the diffusion process. The thermo-diffusion in elastic solids is due to coupling of temperature, mass diffusion and strain fields addition to heat and mass exchange with the environment. Nowacki (1974, 1976) developed the theory of coupled thermoelastic diffusion. The recent development of generalized theory of thermoelastic diffusion by Sherief et al. (2004) allows the finite speed of propagation of thermo-diffusive waves. Sharma (2007) discussed the propagation of plane harmonic waves in generalized thermo-elasto-diffusive solid. Kumar et al. (2014) studied the propagation of Rayleigh waves in a homogeneous isotropic micro-stretch generalized thermoelastic diffusion solid half-space.
The phenomenon of wave reflection and refraction is a fundamental topic in many fields such as seismology, geophysics, earthquake engineering, non-destructive evaluation, etc. Jeffreys (1930) and Gutenberg (1944) considered the reflection of elastic plane waves at a solid half space. Knott (1899) derived the general equations for reflection and refraction of waves at plane boundaries. Singh (2005) discussed the reflection of
P
andSV
waves from the free surface of an elastic solid with generalized thermo-diffusion. Singh (2006) studied the reflection of SV waves from the free surface of an elastic solid in generalized thermo-elastic diffusion. Sharma and Sharma (2010) investigated the reflection characteristics of acousto-diffusive waves from the surface of a semiconductor half-space which is subjected to stress free, isoconcentrated and stress free, impermeable conditions. Kumar and Kansal (2012) considered the reflection and refraction of plane waves at the interface of an elastic solid space and a thermoelastic diffusive solid half-space. Bijarnia and Singh (2012) investigated the propagation of plane waves in a transversely isotropic generalized thermoelastic solid half-space with diffusion and they also studied the reflection of these plane waves from a thermally insulated free surface. Kumar et al. (2013) studied the reflection and refraction phenomenon due to plane wave’s incident obliquely at a plane interface between uniform elastic solid half-space and microstretch thermoelastic diffusion solid half-space.Latin American Journal of Solids and Structures 11 (2014) 2141-2170
underlying an inviscid liquid. Sharma and Bhargava (2014) investigated the reflection and transmission of thermoelastic plane waves at an imperfect interface between a thermal conducting viscous-liquid and generalized thermoelastic solid half-space. Sharma, et al. (2008) studied the generalized Rayleigh waves in thermoelastic solids under viscous fluid loading.
Keeping in view the above state facts and applications of reflection/transmission phenomenon in thermoelastic-diffusive solid under the interaction of fluid, the present paper is devoted to discuss the reflection and transmission of plane waves at the interface between such continua. The effects of incident angles, material parameters and fluid loading on reflection and transmission coefficients of various possible waves due to the incident
qP
andqSV
waves have been considered. The analytical results so obtained have been verified numerically and are illustrated graphically.2 FORMULATION OF THE PROBLEM
We consider a homogeneous isotropic, thermoelastic-diffusive solid in the undeformed state initially at uniform temperature
T
0, underlying an inviscid liquid half-space. We take origin of the rectangular Cartesian co-ordinate systemOxyz
at any point on the plane surface (interface) withz
-axis directed normally into the solid half-space, which is thus represented byz
0
as shown in Figure 1. We choose the x-axis along the direction of propagation of waves in such a way that all the particles on the line parallel to the y-axis are equally displaced. Therefore, all the field quantities are independent ofy
-co-ordinate. Further, the disturbances are assumed to be confined to the neighborhood of the interfacez
0
and hence vanish asz
.Liquid
Solid
Figure 1: Geometry of the problem.
1
2
3
4
5
O
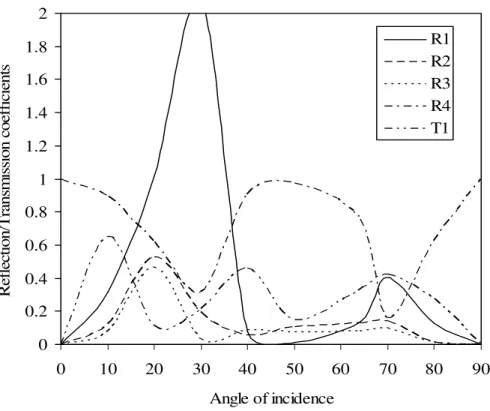
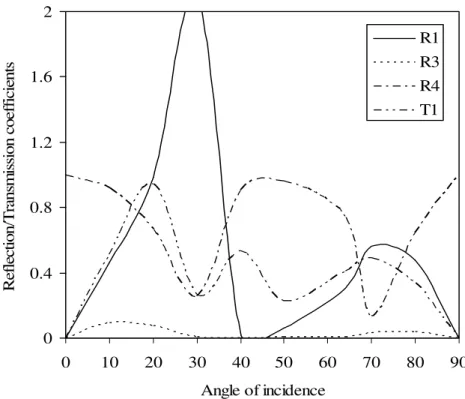
qP or qSV (incident)
qSV (reflected)
T‐mode (reflected) qP (reflected) qP (transmitted)
Z
X
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
The basic governing field equations of motion, heat conduction equation, mass equation and constitutive relations for a solid medium, in the absence of heat sources and body forces, are given by Sherief et al. (2004)
u
C
T
u
u
2
1
2
)
(
(1)
0
)
(
)
.(
)
(
0 1 0 0 0 02
T
C
T
t
T
T
u
t
u
aT
C
t
C
K
e
(2)
0
)
.
(
)
(
1
2 2 21
2
T
b
a
u
b
C
t
C
Db
C
(3)ij ij
ij ij
kk
ij
e
e
T
C
2
1
2 (4)
3
,
2
,
1
,
,
,
2
e
bC
aT
i
j
k
P
kk (5) where
1
(
3
2
)
T,
2
(
3
2
)
C,Here
u
(
x
,
y
,
z
,
t
)
(
u
,
v
,
w
)
,T
(
x
,
y
,
z
,
t
)
andC x y z t
( , , , )
are the displacement vector, temperature change and mass concentration respectively. Here
T,
C are coefficients of linearthermal expansion and linear diffusion expansion;
,
are Lame’s parameters;
is the mass density;K
is the thermal conductivity;D
is the mass diffusion coefficient;a
andb
are thermo-diffusive and thermo-diffusive constants;t
0 andt
1 are thermal and mass flux relaxation times andC
e isthe specific heat at constant strain. The quantity
ij is the Kronecker’s delta withj
1
for Lordand Shulman (LS) theory (1967) and
j
2
for Green and Lindsay (GL) theory (1972). The superposed dot notation is used for time differentiation.The basic governing equations for inviscid fluid (liquid) medium are given by
L L L L L
L
u
T
u
(6)
L V L
L
L
u
C
T
T
0
.
(7)
where
L
3
L
;
L is the bulk modulus;
L and
are the density and coefficient of volume thermal expansion;u
L
(
u
L,
0
,
w
L)
is the velocity vector andT
L is the temperature deviation in the liquid temperature from its ambient temperatureT
0.In order to facilitate the analysis, we define the following dimensionless quantities
,
,
,
,
,
,
0 0
0 1
1
T
T
C
C
T
T
t
c
z
Z
c
x
X
LL
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
,
,
,
,
0 1 1 0 1 1 0 1 1 0 1 1T
w
c
W
T
u
c
U
T
w
c
W
T
u
c
U
L L L L
(8),
,
,
,
,
0 0 1 12 1 2 2 2 1 2 2 2 0 1
t
t
c
c
c
c
T
L L ij ij
,
,
)
2
(
,
)
2
(
0 2 2 2 0 2 1 L V L L L C e Tc
T
b
C
T
1 0 1 0 2 0 00
,
,
,
L eT
C
C
b
aT
b
C
aC
a
where L L L be
c
c
c
Db
c
w
K
C
2
2
2 2
1 2
1
,
2
,
,
~
,
)
2
(
.Here
is the characteristic frequency,
Tand
C are thermo-mechanical coupling constant and mass concentration of the solid respectively,c
1,
c
2 are respectively the longitudinal andshear wave velocities in the solid half-space,
L is the thermomechanical coupling andc
L is thevelocity of sound in the fluid.
Upon using quantities (8) in equations (1)-(7), we obtain
U
U
U
2(
1
2)
(9)
0
)
(
)
(
)
(
0 0 02
TU
U
a
(10)
0
)
(
)
(
~
2 21
2
w
b cU
b
(11) ij ij i j j i ijij
U
U
U
(
1
2
2)
2(
,
,)
(12)
U
b
P
C
ij
(13)
L L L
L
U
U
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
where
L and the operator
and
2are again designated to have their usual meaning in
the changed variables
The scalar point potential functions
and
L and vector point potential functions
and
L have been defined through the relations given below:0
.
,
U
0
.
,
L L LL
U
(16)where
U
(
U
,
0
,
W
)
,U
L
(
U
L,
0
,
W
)
In case
(
0
,
,
0
)
and
L
(
0
,
L,
0
)
, the above equations provide usZ X
U
,
,
,W
,
Z
,
XX L L
U
,
,W
L
L,
Z (17)Upon introducing expressions (17) in equations (9)-(15), and noting that
L
0
for inviscid fluid, one obtains
2
2
1
(18)
2(19)
0
)
(
)
(
)
(
0 02 0
2
T
a
(20)0
)
(
~
4 21
2
w
b
C
b
(21)0
)
1
(
2
2
L L
L
L
(22)L L L
L
)
1
(
(23)The equation (18) corresponds to decoupled shear motion which remains independent of temperature and mass concentration changes.
3 BOUNDARY CONDITIONS
The boundary conditions to be satisfied at the solid-liquid interface (
Z
0
) are given byp
ZZ
,
XZ
0
,W
W
L (24)
0
)
(
,
Zh
L (25)0
,z
(26)
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
where
h
is Biot's constant,h
0
corresponds to the thermally insulated boundary and
h
refers to the isothermal one and the pressure (p
) of the liquid is givenby
p
L
L
2
4 SOLUTION OF THE PROBLEM
We assume wave solutions of the form
)}
cos
sin
(
exp{
}
,
,
,
,
{
}
,
,
,
,
{
L
A
B
C
D
E
ik
X
Z
c
(27)
where
1
c
c
c
,
,
1
c
k
k
are the non-dimensional phase velocity, frequencyand wave number of waves respectively. The primes have been suppressed for convenience. Upon using solution (27) in equations (18)-(23), one obtains a system of algebraic equations in unknowns A, B, C, D and E. The condition for the existence of non-trivial solution of this system of equations on simplification, provides us
)
5
,
4
,
3
,
2
,
1
(
2 2
2
j
a
k
j j
(28)
where
)
1
/(
)
)(
1
(
)
1
)(
1
[(
~
1
1 02
1
w
ba
b
ab
T a Ca
(29)
)
1
/(
)]
1
(
1
[
~
)
1
(
1 00 2 2 2
1
a
a
b
w
b T Ca
)
1
/(
~
1 0 2 3 2 2 2
1
a
a
w
b Ca
2 2 4
1
a
,)
1
(
1
2 2 5
L L
a
1 0 0
i
, 11 1
i
,
a
Cb
In the absence of mass diffusion
a
0
2, the quantitiesa
i2(
i
1
,
2
,
3
,
4
,
5
)
defined in equation(29) become
0 2 3 2 1 0
2 3 2 1 1 2
2
,
1
(
1
)
,
~
w
a
a
a
a
a
b T , 22 4
1
a
,)
1
(
1
2 2 5
L L
a
(30)
Latin American Journal of Solids and Structures 11 (2014) 2141-2170 0 2 3 1 2 2 2 1
,
~
,
1
a
w
a
a
b , 2 24
1
a
, 52 21
La
(31)The equation (30) and (31) will be used for reductions in the following analysis.
5 REFLECTION AND TRANSMISSION AT SOLID-LIQUID INTERFACE
In this section we shall discuss reflection and transmission of waves at the interface of thermo-elasto-diffusive solid and inviscid fluid for
qP
-wave andqSV
-wave incidence cases.5.1. Quasi longitudinal
(
qP
)
-wave incidenceSuppose that a
qP
-wave is incident at the interface from the solid half-space. Then the total wave fields after reflection and transmission of waves from the interface are given by)
exp(
)}
cos
sin
(
exp{
)}
cos
sin
(
exp{
3 1 1 1
i
Z
X
ik
A
Z
X
ik
A
j j j j rj i r i
(32)
exp{
4(
sin
4cos
4)}
exp(
)
4
r
A
rik
X
Z
i
(33)
)
exp(
)}
cos
sin
(
exp{
)}
cos
sin
(
exp{
3 1 1 1 1
i
Z
X
ik
A
S
Z
X
ik
A
S
j j j j r j i r i j
(34))
exp(
)}
cos
sin
(
exp{
)}
cos
sin
(
exp{
3 1 1 1 1
i
Z
X
ik
A
V
Z
X
ik
A
V
j j j j r j i r i j
(35)
5exp{
5(
sin
5cos
5)}
exp(
)
Lt
A
ik
X
Z
i
(36)
S
LA
5exp{
ik
5(
X
sin
5Z
cos
5)}
exp(
i
)
Lt
(37)where
S
jS
j2
,V
jV
j2
,S
LS
L2
)
1
(
2jj
j
V
a
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
)
1
(
LL L
S
3
,
2
,
1
],
)
1
[(
/
]
)
1
(
1
[
2 2 12
b
a
a
b
a
w
j
V
j j
a j
j
b (38)In the absence of mass diffusion(
a
0
2), we have
2
,
1
3
,
1
,
0
j
j
V
j ,
2
,
0
3
,
1
),
1
(
2j
j
a
S
j j (39)In the absence of mass diffusion and thermal variations
(
a
0
2,
T
0
)
, we have
2
,
1
3
,
1
,
0
j
j
V
j ,
2
,
1
,
0
3
),
1
(
0j
j
S
j
(40)
Upon using equations (32)-(37) and employing the boundary conditions (24)-(26), one obtains a system of five coupled algebraic equations (A.1)-(A.5) given in the Appendix.
Since all the waves, incident, reflected or transmitted must be in phase at the interface
Z
0
for all values ofX
and
, therefore the equations (A.1)-(A.5), lead to
sin
1
k
k
1sin
1
k
2sin
2
k
3sin
3
k
4sin
4
k
5sin
5 (41)
The equation (41) with the help of equation (28) implies that
sin
1
a
a
1sin
1
a
2sin
2
a
3sin
3
a
4sin
4
a
5sin
5 (42)
This is the modified form of the Snell’s law, which in the absence of thermal, mass diffusion, viscosity and fluid fields
(
0
,
L
0
L,
a
0
2,
L
0
)
, becomes4 1
sin
sin
This implies that
2 4 1
1
sin
sin
c
c
(43)Latin American Journal of Solids and Structures 11 (2014) 2141-2170
The system of equations (A.1)-(A.5) with the help of equation (41) can be expressed as
B
AZ
p
(44) where
A
,Z
p andB
are defined in the Appendix.Solving the system of equations (44), the amplitude ratios
R
kqP(
k
1
,
2
,
3
,
4
)
andqP
T
1 are obtained as
11
qP
R
,
22
qP
R
,
33
qP
R
,
4 4qP
R
,
51
qP
T
(45)
where
A
has been defined in equation (A.9) of Appendix and
i(
i
1
,
2
,
3
,
4
,
5
)
can be obtained from
by replacing first, second, third, fourth and fifth column by
51 1 41 1 31 21
11
a
a
S
a
V
a
a
, respectively.For the normal
(
0
0
1
5)
and grazing(
90
0
1)
incidence, the relation (45) reduces to
11
qP
R
,
22
qP
R
,
33
qP
R
,
4 4qP
R
,
51
qP
T
and
1
1
qP
R
,R
qPR
qPR
qPT
qP1 4 3
2
0
(46)
respectively. The quantities
and
i(
i
1
,
2
,
3
,
4
,
5
)
have been defined in equation (A.9) of Appendix.Thus for the grazing incidence, the reflected
qP
wave annihilates the incidentqP
wave and there is no reflection or transmission of other waves through the interface.In the absence of mass diffusion
(
a
0
2)
, the non vanishing amplitude ratios are
ˆ
ˆ
1 1
qP
R
,
ˆ
ˆ
2 3
qP
R
,
ˆ
ˆ
3 4
qP
R
,
ˆ
ˆ
4 1
qP
T
(47)Here the quantities
ˆ
,
ˆ
i(
i
1
,
2
,
3
,
4
)
are defined by equation (A.9) in the Appendix.In the absence of mass diffusion and thermal fields
(
a
0
2,
T
0
)
, the non vanishing amplitude ratios at normal(
0
0
1)
and grazing(
90
0
1)
incidence ofqP
wave, are given by1 1 1
c
c
c
c
R
L L
L L qP
, qP qPR
R
3
0
4 ,1 1
2
c
c
c
T
L L
L qP
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
1
1
qP
R
, qP qP qPT
R
R
3
4
0
1(48)
respectively. The relations (48) are in complete agreement with the corresponding equations of Achenbach (1973). Clearly
qP
wave is reflected as well as transmitted in case of normal incidence, however, for grazing incidence the reflectedqP
-wave annihilates the incident one.5.1.1 Quasi longitudinal
(
qP
)
-wave incidence at free surfaceIn case the liquid media is absent
(
L
0
)
, the amplitude ratios for stress free, insulated and stress free, isothermal thermoelastic half-space are, respectively, given byL
qP
S
i
a
a
a
R
3 3 3 11 241
cos
2
1
L
qP
S
i
a
a
a
R
1 1 1 11 24 3cos
2
(49)L
qP
a
i
S
a
a
a
S
a
a
a
R
2
11{
1 1cos
1 41 23 3 3cos
3 43 21}
4
and
L
qP
S
a
a
a
a
S
a
a
R
1 13 24 23 14 3 11 241
)
(
2
1
L
qP
S
a
a
R
1 21 143
2
(50)
L
qP
S
a
a
S
a
a
R
42
1 21 13 3 11 21
where
)
(
cos
)
(
cos
1 13 24 23 14 3 3 3 11 24 21 141
1
i
a
a
a
a
a
S
i
a
a
a
a
a
S
L
(51)
)
(
)
(
13 24 23 14 3 11 24 21 141
a
a
a
a
S
a
a
a
a
S
L
(52)
In the absence of mass diffusion, fluid and thermal fields
(
a
0
2,
T
0
L)
, the non-vanishing amplitude ratios are4 2 4 1
2
4 2 4 1
2 1
2
cos
2
sin
2
sin
2
cos
2
sin
2
sin
qP
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
4 2 4 1
2
4 1
2 4
2
cos
2
sin
2
sin
2
cos
2
sin
2
qP
R
(53)
Relations (53) are in complete agreement with the corresponding equations as in Achenbach (1973). In case of both normal
(
0
0
1)
and grazing(
90
0
1)
incidence, the relations (53) provide us1
1
qP
R
,R
4qP
0
(54) Thus, the incidentqP
-wave is reflected asqP
-wave without change in phase in case of normal incidence and the reflectedqP
-wave annihilates the incidentqP
-wave for grazing incidence one. It may be noted from the above analytical expressions for the reflection/transmission coefficients that the characteristics of reflected and transmitted waves depends on material parameters and incidence angle in addition to thermal variation and fluid loading effects.5.2 Quasi transverse
qSV
-wave incidenceNow consider the reflection and transmission of a plane
qSV
-wave for similar conditions on the boundary as in Section 5.1 above. The total displacement field in this case is given by
exp{
(
sin
cos
)}
exp(
)
)}
cos
sin
(
exp{
4 4
4 4
4
4
i
Z
X
ik
A
Z
X
ik
A
ir
i
r,
r,
r,
L
Lt,
L
Lt (55)where
r,
r,
r,
Lt,
Lt are defined in equations (32)-(37).Upon using expressions (55) in the boundary conditions (24)-(26), at the surface
Z
0
and assuming that all the incident, reflected or transmitted waves are in phase at this surface for all values ofX
and
, so that
sin
4
k
k
1sin
1
k
2sin
2
k
3sin
3
k
4sin
4
k
5sin
5 (56)
we obtain a system of five coupled algebraic equations given as
1
B
AZ
s
(57)
Where
* 1 51
411 34 24 14
1
a
a
a
S
a
V
a
B
,
qSV qSV qSV qSV qSV
s
R
R
R
R
T
Z
1,
2,
3,
4,
1
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
11
~
qSV
R
,
22
~
qSV
R
,
3 3~
qSV
R
,
44
~
qSV
R
,
51
~
qSV
T
(58)
where
1
( 1, 2, 3, 4)
k
r qSV k
i
A
R k
A
and
1
5 1
qSV
i
A
T
A
are amplitude ratios of the reflected andtransmitted waves. Here, the quantities
~
i(
i
1
,
2
,
3
,
4
,
5
)
used in equation (58) can be obtainedfrom
by replacing first, second, third, fourth and fifth column by
1 51
* 41 1 34 24
14
a
a
S
a
V
a
a
respectively.In case of both normal
(
0
0
4
5)
and grazing(
90
0
4)
incidence, the relation (58) reduces to
1
4
qSV
R
,qSV qSV
qSV qSV
T
R
R
R
1
2
3
0
1 (59) Thus the shear (qSV
) wave is reflected asqSV
-wave in case of normal incidence and thereflected (
qSV
) wave annihilates the incident wave for grazing incidence.In the absence of mass diffusion
(
a
0
2)
, the non vanishing amplitude ratios are
ˆ
ˆ
1 1
qSV
R
,
ˆ
ˆ
2 3
qSV
R
,
ˆ
ˆ
3 4
qSV
R
,
ˆ
ˆ
4 1
qSV
T
(60)
Here, the quantities
ˆ
i(
i
1
,
2
,
3
,
4
)
used in equation (60) can be obtained from
ˆ
by replacingfirst, second, third and fourth column by
*
41 1 34 2414
a
a
S
a
a
respectively.In the absence of mass diffusion and thermal fields
(
a
0
2,
T
0
)
, the amplitude ratios at normal(
0
0
1)
and grazing(
90
0
1)
incidence ofqSV
wave, are given byqSV qSV
qSV
T
R
R
1
3
0
1 , 4
1
qSV
R
(61)
Thus only shear (
qSV
) wave is reflected asqSV
-wave in case of normal incidence and the reflected (qSV
) wave annihilates the incident wave for grazing incidence case. The other waves do not reflect or transmit in either case.5.2.1 Quasi transverse
qSV
-wave incidence at the free surfaceIn case
(
L
0
)
, the amplitude ratios for stress free, insulated and stress free, isothermal thermoelastic half-space are, respectively, given by
L
qSV
i
S
a
a
a
a
a
S
a
a
a
R
1 1 1 13 24 23 14 3 3 3 14 24 1cos
2
)
(
cos
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
L
qSV
i
S
a
a
a
S
a
a
a
a
a
R
2
1 1cos
1 14 24 1 1cos
1(
11 24 21 14)
3
L
qSV
i
S
a
a
a
S
a
a
a
S
a
a
a
a
a
R
1
2
1 1cos
1 13 242
3 3cos
3 11 24 1 1cos
1(
11 23 21 13)
4
(62)
and
L
qSV
S
a
a
a
a
S
a
a
R
1 13 24 23 14 3 14 241
2
)
(
L
qSV
S
a
a
S
a
a
a
a
R
2
1 24 14 1(
11 24 21 14)
3 (63)
L
qSV
S
a
a
S
a
a
S
a
a
a
a
R
1
2
1 24 132
3 11 24 1(
11 23 21 13)
4
where
L and
Lare defined in equations (51) and (52) respectively.In the absence of mass diffusion, fluid and thermal fields
(
a
0
2,
T
0
L)
, the non vanishing amplitude ratios are3 2 3 1
2
3 1
2
cos
2
sin
2
sin
4
sin
qSV
R
3 2 3 1
2
3 2 3 1 2
4
2
cos
2
sin
2
sin
2
cos
2
sin
2
sin
qSV
R
(64)
Equations (64) are in agreement with the corresponding equations as in Achenbach (1973). In case of both normal
(
0
0
4)
and grazing(
90
0
4)
incidence ofqSV
wave, the expressions for reflection coefficients in equation (63) provide us0
1
qSV
R
,R
4qSV
1
(65) Thus only shear wave is reflected asqSV
wave without any change of phase in case of normal incidence and reflectedqSV
-wave annihilates the incidentqSV
wave in case of grazing incidence. It is noticed that the reflection/transmission characteristics of waves depends upon material parameters and incidence angle in this case too.6 TOTAL REFLECTION
Latin American Journal of Solids and Structures 11 (2014) 2141-2170
sin
4
k
k
isin
i(
i
1
,
2
,
3
,
4
)
(66)
Here the quantities
a
i2(
i
1
,
2
,
3
,
4
)
are complex and so does at the wave numbers)
4
,
3
,
2
,
1
(
2
i
k
i are also complex. Thus the phase velocities(
c
i
(
/
k
i)
i
1
,
2
,
3
,
4
)
of thesewaves are complex and the waves becomes attenuated in space. If we take
4
,
3
,
2
,
1
,
1 1
1
j
Q
i
V
c
j j
j (67)
where
k
j
j
iQ
j,
j
/
V
j andQ
j(
j
1
,
2
,
3
,
4
)
are real quantities. ThenV
j,Q
jrespectively represent phase speed and attenuation coefficients of these waves. Upon using relations (67) in equation (66), we get
4sin
1sin
1
2sin
2
3sin
3
4sin
4
1 1
sin
Q
Q
2sin
2
Q
3sin
3
0
(68)
Because
Q
4
0
, therefore4 4
k
,
4 ,V
4
c
4, 41 4 1
sin
sin
V
V
j jNow upon using equations (66)-(68), the potential function
r given in equation (32) can be rewritten as
31
2 2 2
4
(
sin
sin
)}
exp(
)
exp{
}
exp{
j
j j
rj
r
A
Q
Z
i
k
X
Z
V
i
Now for
qP
andqSV
wavesV
2
V
12 2 2 2 1
2
V
V
andsin
increases to the value 1 1
V
first for
2
0
. Ifsin
V
1sin
c1
, then
c is called critical angle. For
cthefactor
2 2
12
sin
V
becomes purely imaginary. In the absence of thermal field the critical angle
c for elastic wave is obtained whenV
1
1
, so that
1
sin
c . Thus in the presence of
thermal field, the value of critical angle increases as
V
1
1
or
1
. For 1 2 1 1sin
V
V
, we have
21
4 2
2 2
4
sin
}
]
exp{
(
sin
)}
{
exp[
j
j j
rj
r
A
Q
k
V
Z
i
k
X
(69)
Thus the thermal part and elastic part of reflected P-wave propagates horizontally in
X
-direction and these quantities decay exponentially with depth.
Moreover, we have also considered the relation
a
1sin
1
sin
, which shows that
1is realonly if
c, where
1
1sin
a
c