Ising Meets Ornstein and Zernike, Debye and Hukel,
Widom and Rowlinson, and Others
Ronald Dikman
Departamentode Fsia, ICEx,
UniversidadeFederalde MinasGerais,
30123-970BeloHorizonte-MG,Brasil
Reeivedon20August,2000
The name Ising has ometo stand not only for a spei model, but for an entire universality
lass - arguably the most important suh lass - in the theory of ritial phenomena. I review
severalexamples,bothinandoutofequilibrium,inwhihIsinguniversalityappearsorispertinent.
The \Ornstein-Zernike" onnetion onerns a thermodynamially self-onsistent losure of the
eponymousrelation,whihliesatthebasisofthemoderntheoryofliquids,asappliedtotheIsing
lattie gas. DebyeandHukelfoundedthe statistialmehanisofioni solutions,whih,despite
thelong-rangenatureoftheinteration,nowappeartoexhibitIsing-likeritiality. Themodelof
WidomandRowlinsoninvolvesonlyexluded-volumeinterationsbetweenunlikespeies,butagain
belongsto theIsinguniversality lass. Far-from-equilibriummodelsofvotingbehavior,atalysis,
andhysteresisprovidefurtherexamplesofthisubiquitousuniversalitylass.
I Introdution
Sine the playful title of this review might generate
onfusion, let me start by saying that I have no idea
whetherornotErnstIsingatuallymetanyoftheother
sientistsmentioned. Theworldlinesintersetinghere
belong to models and theories,not to persons, realor
imagined! MuhasthenameGalileohasometodene
aspeikindofrelativity,Hamiltonakindof
dynam-is, andGauss adistribution,so\Ising" isnow
indeli-blyassoiatedwithaspeikindofritialbehavior,a
\universalitylass"inthefamiliar jargonof
renormal-izationgrouptheory. Thelattertellsus(asisborneout
byexperiment,analysis,andsimulation),thatthe
fa-torsdeterminingsalingpropertiesintheneighborhood
of the ritial point are few, and pertain to very
gen-eralpropertiesofthesystem,suhasitsdimensionality
and that oftheorder parameter,rangeand symmetry
ofinterations. Forhistorialreasons,thelassdened
byasalarorderparameter,,short-rangeinterations,
isotropy,andsymmetryunderinversion(! inthe
abseneofanexternaleld),isommonlyknownasthe
Ising universalitylass. Whileweshould expet many
systemstofall inthislass,mostofthepresentartile
isdevotedtoexampleswhosemembershipissurprising
or ontroversial.
My rst example, self-onsistent Ornstein-Zernike
theory(Se. II),isnotabona-dememberoftheIsing
lass, butis,in asense,makinganimpressiveeortto
beone! Critialityineletrolytesolutions,disussedin
Se. III,remainsontroversial,thoughreent
theoreti-aland experimental studiessupport Ising-like
behav-ior. Wewill seethatthesimplest lattieversionofthe
well-knownprimitivemodelmayexhibitalineof
Ising-likeritialpoints. WeareusedtothinkingoftheIsing
model as possessing two relevantvariables (or saling
elds),thetemperatureandtheexternalmagnetield.
TheWidom-Rowlinson model(Se. IV) (inits
lattie-gasversion)illustratesthepossibilityofIsingritiality
in asystemwith no temperature to speakof. Having
dispensedwith temperature, we proeed in Se. V to
the more radial step of eliminating the Hamiltonian
(andthermodynamis) altogether, in several
far-from-equilibriumstohastiproesses. Theguidingpriniple
ofsymmetry againpermits one to understand the
ap-pearene of Ising ritiality in these systems. A brief
summaryisprovidedin Se. VI.
II Self-Consistent
Ornstein-Zernike Theory for the Ising
Model
Ornstein-Zernike theory [1℄ represents an important,
earlyhapterinthetheoryofritialphenomena,andis
stillwidelyusedtointerpretdatafromsattering
exper-iments. The Ornstein-Zernike relation (OZR),
more-over,servesasthestartingpointformoderntheoriesof
liquids[2℄. This relationintroduesthediret
orrela-tion funtion (r) [4℄ in terms of the totalorrelation
funtion h(r)g(r) 1,where g(r)is the radial
dis-tributionfuntion:
h(r)=(r)+ Z
dr 0
(r)h(jr r 0
j): (1)
Hereisthenumber-density. Of ourseonemustnow
supply a losure, i.e., a seond relation between (r),
theintermoleularpotentialw(r),and(inertainases)
h(r) itself. This, in general, an be done only in a
heuristimanner,asforexampleinthemean-spherial
approximation, (r) = w(r)=k
B
T, or the more
so-phistiatedPerus-Yevikorhypernettedhainlosures
[2,3℄. Surprisingly,thesesimplelosuresleadin many
ases to good preditionsfor h(r), and for
thermody-nami properties, viaone of the standard, exat
rela-tions (the so-alled virial, ompressibility, and energy
routes) involving h(r). This is remarkable, sine
nei-ther the OZR nor the losure inlude any
thermody-namiinput. TheOZformalismand theresulting
`in-tegralequations' for h(r) are ouhed purely in terms
oforrelations,withoutrefereneto afreeenergy.
Theabsene of thermodynami input beomes
ev-ident when one ompares the preditions from
dier-entroutes. Thus thePerus-Yevik losureyields two
dierent equations of state for the hard-sphere uid,
depending on whether the link to thermodynamis is
madeusingthevirialortheompressibilityroute. Suh
inonsistenies suggest a new strategy for losing the
OZR, in whih we use a thermodynami relation,
ex-pressing equality of (for example) the pressure ev
alu-ated viadierentroutes, to nd aseond relation
be-tween h, , and w. Suh an approah was proposed
over twenty years ago by Hye and Stell [5℄, but
de-tailed numerial implimentations have appeared only
in the last few years. The rst suh appliation was,
naturally, to thethree-dimensionalIsingmodel,whih
weoutlinehere. (Fordetailstheinterestedreadermay
onsultRefs. [6℄and[7℄.)
We onsider the Ising lattie gas with
indepen-dent variables (number of partiles per site) and
1=k
B
T. The interpartile potential w(r) is zero
forr >1, -1forr =1(anattrativenearest-neighbor
interation) and innitefor r =0. The latterimplies
the `ore-ondition' h(0) = 1, expressing the simple
fat that twopartiles may notoupy thesame site.
On a lattie the virial route, whih involves the
spa-tial derivative of the potential, does not exist.
Con-sistenybetweenthetworemainingroutes,energyand
ompressibility,isembodiedintherelation
2
(u)
2
=
2
(p)
; (2)
pressure. Theenergyrelationis
u= 1
2
q(1+h
1
); (3)
where h
1
denotes thetotal orrelationfuntion at the
nearest-neighborseparation,and q isthe oordination
number. Theinverseompressibilityisrelatedto via
(p)
=1 ~(0) (4)
where~denotestheFouriertransform. Thediret
or-relationfuntionis,ineet,denedbytheOZR,whih
reads,onlattie,
h
i =
i +
X
j2L
i j h
j
; (5)
(the sumis overthesites oflattie L). This relation,
or,morespeially,itsFourierrepresentation,
1+ ~
h= 1
1 ~
; (6)
enables us to nd h given . Sine we already have
h(0)= 1,theaboverelations, inorporating
thermo-dynami onsisteny, suÆe for determining one free
parameter, as a funtion of and . At this stage
weintrodue theunique approximation of ourtheory,
whihistoset(r)0forr>1,i.e.,beyondtherange
of the interation. This denes what we allthe
self-onsistentOrnstein-ZernikeapproximationorSCOZA.
After some manipulations we obtain a nonlinear
par-tialdierentialequationfor (1)=
1
(;) ((0) is
ef-fetively xedby theoreondition). Integratingthis
PDEnumeriallyeventuallyyieldsh(r)andall
thermo-dynamipropertiesofthelattiegas.
The results ompare remarkably well against the
bestnumerial(seriesexpansion)estimatesforthe
lat-tie gas [6, 7℄. We nd the ritial temperature from
theonditionofadivergingompressibilityat=1=2
(belowT
,inniteompressibilitysignalsthespinodal);
theoexisteneurveisonstrutedin theusual
man-ner. Espeially impressivearethe preditionsfor
rit-ialparameters,(Ref.[6℄);SCOZAreproduesthebest
seriesestimatestowithin 0.2%.
Can SCOZA predit ritial exponents? Its
ther-modynami preditions are so aurate that eetive
exponents (i.e., derivatives of the ompressibility,
or-der parameter, et., with respet to temperature) are
in reasonableagreementwith Isingmodel valuesnear,
but notasymptotiallyloseto, theritialpoint. We
reallyshouldnotexpetSCOZAtoreprodueIsing
rit-ial exponents: (r) is notzero forr > 1in theIsing
model. In partiular, it develops a power-law tail at
the ritial point. The atual ritial behaviorof the
SCOZA-lattiegasisunusual[7℄: forT >T
the
expo-nents arethose ofthe spherialmodel: =2, Æ =5,
and = 1(the speiheat doesnotdivergeat T
the three-dimensionalIsing model. Below T
, SCOZA
yields a new set of exponents: 0
= 7=5, 0
= 7=20
and 0
= 1=10, onsiderably better than mean-eld
or spherialmodel values.
Clearlythe wayto improveSCOZAis torelax the
trunation of (r). But even with its urrent
limita-tions,SCOZArepresentsthersttheorytoyield
glob-allyauratethermodynamiandstruturalproperties
for auid model. It has been applied with suessto
o-lattie uids[11℄, anduids inadisorderedmatrix
[12℄,andpromissestobeomeanimportanttoolinthe
study ofliquids.
III Ising Universality and the
Primitive Model of
Ele-trolytes
Theritialbehaviorofeletrolytesolutionsremainsa
hallengingarea,bothexperimentallyandtheoretially.
Experimentsonvariousionisolutionshaveyielded
ei-ther mean-eld like ritial exponents [13℄, Ising-like
exponents[14℄,orarossoverfrommean-eldto
Ising-likebehaviorasonenearstheritialtemperature[15℄.
Insomeasestherossoverfrommean-eldtoIsing
ex-ponentsoursatareduedtemperaturemuhsmaller
thanthat typiallyseenin non-Coulombliquids.
Theoretialstudieshavetendedtofousonthe
sim-ple system introdued by Debye and Hukel in 1923
[16℄,ommonlyknownastherestritedprimitivemodel
(RPM).Here thepositiveandnegativeionsare
repre-sentedbyhardspheresofdiameter,ontainingpoint
harges q at their enters. The solvent is not
on-sidered expliitly; its eet is represented solely by
the dieletrionstant that enters theexpression for
the eletrostati potential. While learly a minimal
model for ioni solutions, the RPM already presents
great diÆulty to theory and simulation. Debye and
Hukel'sanalysisofthedilute,high-temperatureregime
led to the fundamental result that eletrostati
in-terations are sreened on a length sale 1
, where
2
=4q
2
=. (Theeetiveinteration orpotential
of meanfore takesonaYukawaform /e r=
=r.)
Whatsortofphasediagramshouldweexpetforthe
RPM?Thehard-spherepartofthepotentialalonewill
lead to auid-solid transition. At high temperatures
(T
k
B T=q
2
1) we expet paking
onsidera-tionstodominate,sothatthehigh-densitystrutureis
FCCorHCP.Butsinethesestruturesare
inompati-blewithantiferromagnetiorder(i.e.,positiveand
neg-ativehargesoupyingdistintsublatties),weshould
expetastrutural phasetransitionto abipartite
lat-tie (presumably BCC) as welower the temperature,
at highdensity.
The opposite orner of the T plane, low
tem-we nd the ritial point in simple uids. Sine the
RPM laks the short-rangeattration that drives the
liquid-gastransition,itisnotimmediatelyobviousthat
itshouldexhibitsuhatransition. Nevertheless,Stell,
Wu,and Larsen,using liquid-statetheory,reahedthe
onlusion that the RPM has aliquid-gas oexistene
urve with a ritial point [17℄, but with a ritial
density muh lowerthan for asimple argon-likeuid.
MonteCarlosimulationsonrmtheseonlusions,but
onlyin the last fewyearshavethestudies of dierent
groupsonverged towardommon valuesfor the
riti-aldensityandtemperatureoftheRPM[18-20℄. Given
thediÆultyin simplyloatingthe ritialpoint, itis
notsurprisingthaturrentsimulationsshedlittlelight
onitsnature.
On the theoretial side, however, there has been
onsiderabledisussionofRPMritiality,pointing
to-ward Ising-like behavior [21-23℄. This seems at odds
withonventionalwisdom(Isinguniversalityfor
short-range,and mean-eld behaviorfor long-range
intera-tions),butmaybeunderstoodintuitivelyasfollows. At
thelowtemperaturesofinterest(notethatT
0:05),
theCoulombiinteration stronglysuppresses
harge-density utuationsonsaleslargerthan; ions
asso-iateinto pairsand largeraggregates. Thus the
ee-tiveinteratingunits are notindividual ions but
lus-tersthat are typially neutral(or nearlyso),
interat-ingvia multipolarfores (presumably quadrupole and
higher)thatareofshortrangeand,onaverage,
attra-tive(sinesuhutuationslowertheenergy).
Suhanintuitivepiturendssupportinreent
ex-perimentsshowingIsingritialexponentsatan
ioni-solutionritial point [15℄. What is needed, from the
theoretial standpoint, is an argument that takes us
from the RPM to a ontinuum desription of density
utuations,Æ(r), havingthesameform(up to
irrele-vantterms) asthat fortheIsing model, i.e., theusual
4
eld theory. It is lear, on the other hand, that
the RPM needs to be desribed in termsof a pair of
oupledelds, themassornumberdensityÆandthe
hargedensity . Animportantrststepistheproper
formulation of a mean-eld theory [24, 25℄. Very
re-ently,CiahandStellonstrutedaLandau-Ginzburg
freeenergyfuntional,startingfromthemean-eld
the-ory ofthe RPM,in termsofthe twoelds, Æ and .
Theyndthat integratingoutthehargedensity
u-tuations leads to an eetive eld theory for Æ
hav-ing the expeted 4
form. (Essentially, an attrative
eetiveinteration betweenmass-densityutuations
is mediated by harge-harge orrelations.) Thus the
ruiallink betweentheRPM andIsing-likeritiality
appearstobeathand.
Inlightof thegreat diÆultyof RPMsimulations,
it seems useful to study a lattie restrited primitive
model (LRPM) [26,27℄, sinelattie simulationsoer
ial using a site-oupany matrix, while the Coulomb
potential-with theontributionsfromtheinnite
pe-riodi array of ells suitably aounted for - may be
storedin alookuptable.) Dikman andStell[26℄
on-sideredalattiegasofpartilesinteratingviasite
ex-lusion(multipleoupanyforbidden)andaCoulomb
interationu(r
ij )=s
i s
j =r
ij
,wherer
ij =jr
i r
j jisthe
distaneseparatingthepartiles(loatedatlattiesites
r
i andr
j ),ands
i
=+1or 1isthehargeofpartilei.
(Exatly half thepartiles arepositivelyharged, half
negative. They arerestritedto asimpleubilattie
withperiodiboundaries.)
Atfulloupany,theLRPMmaybeviewedasan
antiferromagnetiIsingmodel withlong-range
intera-tions. One naturally expets a ritial or Neel point
separatingahigh-temperaturephasefrom aphase
ex-hibiting antiferromagneti order. (The Ising analogy
failitates formulation of a mean-eld theory for the
LRPM.)Indeed,itwasprovensometime agothatthe
LRPM on thesimple ubi lattieexhibits long-range
orderat suÆientlylowtemperaturesandhigh
fugai-ties[28℄. Insimulations,wendthatthis pointmarks
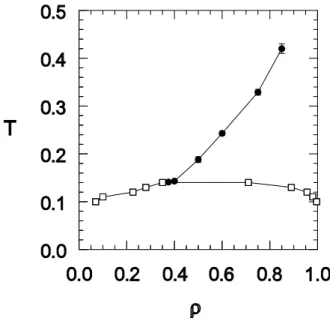
one terminus of a line of ritial points in the T
plane (see Fig. 1). The other end of the ritial line
is a triritial point, whih intersets the oexistene
urve betweenalow-densitydisorderedphase andthe
ordered phase. The Monte Carlo simulations used a
relativelysmalllattie(16 3
sites),suÆienttomapout
the phase diagram by studying the order parameter,
spei heat, and orrelation funtions, but toosmall
toyieldinformationonritialbehavior[26℄.
Figure1. Bestestimatesfortheloationoftheritialline
andoexisteneurveinthelattieRPM(simpleubi
lat-tie).
Thus the simplest version of the LRPM shows
ontinuous-spae RPM, exhibiting a ritial line and
triritialpointratherthanasimpleritialpoint. One
reason for this dierene is that the simple ubi
lat-tiefailitatesantiferromagnetiorder. Itmaybethat
onalattiethat frustratessuhorder(the FCC
stru-ture,forexample),theritialline willdisappear,and
the triritial point beome a ritial point. On the
otherhand,PanagiotopoulosandKumarfoundexatly
this, on the simple ubi lattie, when the hard-ore
exlusionrangeis3timesthelattiespaing[27℄. A
suitably modied versionof theLRPM may therefore
beusefulfor studyingioni ritiality. Thephase
dia-gramappearstobehighlysensitivetohangesinlattie
strutureorshort-rangeinterations,aswasalsonoted
byCiahandStell[25℄.
Evenif theritial line of the simple-ubiLRPM
is notexatlywhatwehad inmind forunderstanding
the o-lattie RPM, its nature, and that of the
asso-iated triritialpoint,are of interest,and potentially
relevanttoothersystemswithCoulombiinterations.
Giventhesymmetryofthesystem,theIsing
universal-itylassagainseemsthenaturalandidate. The(very
limited) simulation data seem to indiate 0:326,
(the3-dIsingvalue),whihshouldinanyaseruleout
a mean-eld typetransition. Clearly larger-sale
sim-ulations andnite-size salinganalysis arein order; a
low-temperatureexpansionfor thefully oupied ase
mightalsoproveuseful. Welosethissetionwiththe
observation that Ising ritiality is ompatible with a
long-rangebareinteration,providedtheeetive
inter-ationsbetweenritialutuationsareofshortrange.
IV Ising Without Temperature:
The Widom-Rowlinson
Model
Inthis setion weagain onsideramodelimported to
the lattie from its original ontinuous-spae
formula-tion. The Widom-Rowlinson (WR) hard-sphere
mix-ture is perhaps the simplest binary uid model
show-ingaontinuousunmixingtransition,andhasbeenthe
subjetofonsiderablestudyregardingitsthermaland
interfaial properties [29, 30℄, as has the Gaussian f
-funtionversionofthemodelintroduedsomewhat
ear-lierbyHelfandandStillinger[31℄. Despitethisinterest,
however,denitiveresultsontheloationandnatureof
theWRritialpointarelaking. Asarststepinthis
diretion,DikmanandStellperformedextensive
simu-lationsofthelattie-gasanalogoftheWRhard-sphere
mixture the Widom-Rowlinson lattie model (WRL)
[32℄.
Intheoriginal WR model,AB pairsinterat via a
hard-spherepotentialwhilstAAandBBpairsare
upied, and in whih nearest-neighbor A-B pairs are
forbidden. Like the WR model, this is evidently an
athermal model (all allowed ongurations are of the
sameenergy),andisharaterizedsolely bythe
densi-tiesofthetwospeiesorbytheorrespondinghemial
potentials
A and
B . (
A =
B =
ofourse,atthe
ritialpoint.)
Despite the absene of a temperature or energy
sale, theWRL model hasaloseaÆnityto theIsing
model. Tosee this,notethattheWRLmaybeviewed
asanextremememberofafamilyofbinary alloy
mod-elswithnearest-neighborinterationsthatarerepulsive
betweenunlike speies (interation energies
AB > 0,
AA =
BB
=0). TheIsing model maybetransribed
into suh a model by identifying up and down spins
with Aand Bpartiles, respetively,yielding a
\lose-paked" alloy that unmixes at the Ising ritial
tem-perature. Allowinga smallfration of vaantsites
re-sults in adilute binary alloy(DBA) with asomewhat
depressedritialtemperature;ontinuingthedilution
proess,onearrivesatamodelwithT
=0. This
zero-temperature terminus of the DBA ritial line is
pre-isely theWRLritialpoint. Theoexistenesurfae
ofthebinaryalloymodelinthe
A ,
B
,Tsolidisshown
shematiallyinFig. 2. Oneisthenledtoaskwhether
the entire ritial line shares a ommon behavior, or
whetheritsharaterhangesatsomepoint. Although
the formeris learlyfavored onthebasis of
universal-ity,aarefulexaminationofthis questionnevertheless
appearsworthwhile.
Figure2. Shematioexistenesurfaeofthebinaryalloy
model. TheIsingmodeloexisteneurveistheintersetion
oftheoexistenesurfaewiththeplaneA+B=1. The
WRL oexistene urve is the intersetion with the plane
T =0.
As shown in Fig. 2, theIsing oexistene urveis
A +
B
=1. For<1wean rossthe ritial
lineinthetemperatureorthedensitydiretion,
main-tainingallthewhileh
A
B =0(
i
isthehemial
potentialofspeiesi). Thus,whileintheWRLthereis
notemperatureperse,
A +
B
isa
temperature-like variable, so that along the symmetry line h = 0,
and in the viinity of the ritial point
, the
sus-eptibility should sale as ' (
)
, the order
parameter,
A
B
'(
)
, (for>
), andso
on[31℄. (Notethatifthehemialpotentialsaretaken
as independentvariables, no\Fisher renormalization"
of ritial exponents is expeted, asit would be if we
workedwithxeddensities[33℄.)
Ref. [32℄ reports Monte Carlo simulations of the
WRL in the grand anonial ensemble, using latties
ofup to 160 2
sites in twodimensions and 64 3
sites in
three dimensions. The algorithm employs three kinds
ofmoves: \ips"(hangeofthestate,A,B,orvaant,
atasinglesite),\exhanges"(betweenanypairofsites
in the system), and ips (A B) of entire lusters
(note that this is always possiblein the WRL sinea
lusterofoupiedsitesisalwayssurroundedbya
bor-derofvaantsites). Theresultsforthereduedfourth
umulant, order parameter, and suseptibility are all
onsistentwithIsing-likebehavior[32℄.
Following the WRL study, a series analysis of a
loselyrelatedontinuummodel,withGaussianMayer
f-funtions, (i.e., the model introdued in Ref. [31℄),
wasreportedbyLaiand Fisher[34℄. This studyagain
provides good evidene for onsisteny with the Ising
universality lass at the ritial point marking phase
separation. More reently, the generalization of the
WRLtoqstates,withinniterepulsionbetweenunlike
nearest-neighborpairshasbeenfound toexhibit
riti-albehavioronsistentwiththeq-statePottsmodel,as
wouldbeexpetedfromsymmetryonsiderations[35℄.
TheappearaneofIsing-likeritialityin athermal
modelsinfatgoesbaktostudiesoflattiegaseswith
nearest-neighbor exlusion (NNE), done in the
mid-1960's[36-38℄. Inthisasethereisonlyasinglespeies
ofpartile,andtheonlyinterationisahard-ore
repul-sionassigninginniteenergyto pairsofpartiles with
separations1, in units ofthe lattie spaing. Ona
bipartitelattiein twoor moredimensions,there is a
ritialdensity abovewhih thepartiles beginto
o-upyoneofthetwosublattiespreferentially,signalling
a ontinuous transition to a state with
antiferromag-neti order. (The NNE lattie gas is in fat losely
related to the zero-temperature line of the Ising
an-tiferromagnet.) Gaunt and Fisher analyzed series
ex-pansions forthe NNE lattie gason various two- and
three-dimensionallatties,andfound 1=8,
suggest-ing, one again, Ising universality [36, 37℄. It would
be worthwhile applying modern series and simulation
methods to the NNE models, to obtain more preise
els mentioned in the present setion (and, indeed, in
the hard-sphere uid), phase separation is driven
ex-lusivelyby entropy maximization. Thus we havethe
apparently paradoxial onlusion that for large
val-ues of the hemial potential, the ordered phase has
an entropy greater thanor equal to that of the
disor-dered phase. This shows that the naiveidentiation
ofentropywith`disorder'isnotalwaysappropriate;
in-terpreting inreasedentropyasgreater freedom seems
moreapt.
V Ising Without Equilibrium:
Voters, Catalysis, and
Hys-teresis
Thepresentsetiononernsfar-from-equilibrium
mod-els, dened by a Markovian dynamis rather than a
Hamiltonian. Sine thetransition rates donot satisfy
detailedbalanewithrespettoanyreasonableenergy
funtion(i.e.,boundedbelow,andwithanitenumber
of many-body terms), these models have no
thermo-dynamiinterpretation. Suhsystemsarenevertheless
widelystudied usingthetoolsofstatistial physis,as
models of, for example, populations, traÆ, atalysis,
and\self-organized"ritiality[40℄. Manyofthese
sys-temsexhibit transitionsbetweenautuation-free
ab-sorbingstate(admittingnoesape)andanativephase
[41,42,43℄. Modelspossessinganabsorbingstate,and,
assoiated with this, a non-negative order-parameter
density, donot exhibit Ising symmetry. Instead, they
fall generiallyin theuniversalitylassofdireted
per-olation,whihplaysaroleanalogoustotheIsinglass
forabsorbing-statephasetransitions[39, 44℄.
The models to be disussed in this setion do,
however, observe Ising symmetry, and belong to the
Ising universality lass. The fat that the Ising lass
uts aross the equilibrium/nonequilibrium boundary
serves to illustrate that phase transitions in
far-from-equilibrium systemsarejust asworthyof thenameas
theirequilibriumounterparts,anddonot,asis
some-timesasserted,representmerelyan\analogy"to\real"
(i.e., equilibrium) phase transitions. Of ourse, one's
outlook will depend on whether oneadopts a
thermo-dynami oramathematialdenition ofaphase
tran-sition. My point is that experiene with perolation,
nonequilibrium models, and deterministi as well as
stohastiellularautomatamakeitnaturaltoregard,
quite generally, any singular dependene of the
prop-erties of a system of many interating units upon its
ontrol parameters as a phase transition. From this
vantage,ritialphenomenarepresentapartiularlass
of singularities (attended, for example,by adiverging
orrelation length and relaxation time), with
equilib-rium ritialpointsrepresentinga partiular, and not
VI The Majority-Vote Model
Theprototypialexampleofanonequilibrium
stohas-ti proess with loal interations, isotropy, and
up-down symmetry is the majority-vote model [44℄. At
eahsiteofalattiethereisa\spin"variable
i =1.
A Markovproessis dened as follows. Ateah step,
a site i is hosen at random, and
i
!
0
i
, whih is
takento beequaltothemajorityofits nearest
neigh-bors (i.e., sgn[ P
jnni
j
℄) with probability p, and to
the minority with probability q = 1 p. (If there is
no majority
i
ips with probability 1/2.) Although
the transition rates do notsatisfy detailed balane, it
is not hard to see that the parameter q plays a role
analogoustemperatureintheequilibrium kinetiIsing
model. (The dynamis of the majority vote model is
akintoGlauberdynamis;therearenoonserved
quan-tities.) For q = 0, \voters" have no independene of
opinionwhatever,andslavishlyfollowtheloal
major-ity. Thisq=0limitdenesthevotermodel,whihhas
twoabsorbingstates,all+1orall-1,justasintheIsing
modelatT =0. (Thevotermodelbelongstoa
univer-sality lass dierent than Ising, the so-alled ompat
diretedperolationlass[45,46℄.) Forq>0butsmall,
the stationary stateexhibits spontaneous
\magnetiza-tion": mh
i
i=m
0
,withm
0
>0. Abovearitial
qvalue(q
'0:075forthesquarelattie[47℄),the
sta-tionarystateis disordered(m=0). (Noordered state
is found in one dimension, just asfor the equilibrium
Isingmodel.)
Thusthephasediagramofthemajority-votemodel
is qualitatively the same as for the standard Ising
model. Conrmationthat theritial behaviorisalso
of theIsing kindomes fromMonteCarlo simulations
reportedbydeOliveira[47℄,whihshowed(forthe
two-dimensional ase), that the exponents, and are
thesameasthoseoftheIsingmodel. Many
nonequilib-riummodelshavenowbeenshowntoexhibitIsing-like
ritialbehavior,indiatingthatthis universalitylass
isequallyrobust,inoroutofequilibrium. Examples
in-ludeafamilyofgeneralizedmajority-votemodels[48℄,
ananisotropimajority-votemodel[49℄,atwo-state
im-munologial model [50℄, and various two-temperature
Ising models [51℄. In the latter example, a spin
sys-temevolvesviatwodynamialproesses,for example,
single-spin ips and nearest-neighborexhanges, eah
having its own temperature (see Ch. 8 of Ref. [43℄
forareview). Ising-likeshort-timeritialbehaviorhas
alsobeenestablishedforertainnonequilibriummodels
withup-downsymmetry[52℄.
Theprinipleunderlyinguniversalityofritial
be-havior in these examples appears, one again, to be
symmetry. Whiledetailedrenormalizationgroup
analy-sesarelaking,Grinsteinetal. havearguedthatmodels
obeying up-down symmetry and exhibiting a
astandardkinetiIsingmodel[53℄. Weanseethisas
follows: ifsuhadesriptionexists,thenina
Langevin-like equation for the order parameter density m(r;t),
only odd powers of m may appear (up-down
symme-try). Gradienttermsareruled out by isotropy, so the
lowest-orderderivativetermwillber 2
m. Generially,
thetransitionto anorderedstatewillbeontrolledby
theoeÆientaoftheterm/m. (Inmean-eldtheory
this oeÆient vanishesat the ritial point, but in a
full analysisthenonlineartermswill renormalizea
to
anonzerovalue.) Thatis,theorder-parameterdensity
willobey(inthenononservingase)atime-dependent
Landau-Ginzburgequationoftheform
m(r;t)
t
=r 2
m am bm
3
+(r;t); (7)
wherewehavedroppedhigher-orderterms(/m 5
,et.)
sinetheyareirrelevanttoritialbehavior. Thenoise
term(r;t)iszero-mean,andGaussian,with
autoor-relationh(r;t)(r 0
;t 0
)= Æ d
(r r 0
)Æ(t t 0
). In
equilib-rium is of ourseproportionalto temperature. Out
of equilibrium no suh relation exists, but the theory
again predits an Ising-like transition; the deviation
fromequilibriumisfoundtobeirrelevant,nearthe
up-perritialdimension[53℄. Thepriniplethatsystems
withthesamesymmetriesshareaommonritial
be-havior, whether in or out of equilibrium, has reently
been extended to models with Potts-like symmetries
[54,55℄.
Wehaveseenthat avarietyofnonequilibrium
per-turbations preserve the harater of the transition in
spin systems or lattie gases. It is worth notingthat
biasing the hopping rates to favor movement along a
partiular axis (e.g., in a lattie gas with attrative
interationsand aKawasaki-typeexhange dynamis)
breaksanessentialsymmetryoftheIsingmodelby
in-troduingapreferreddiretion. Suh\drivendiusive
systems",astheyhaveometobealled,exhibit
riti-albehavioroutsidetheIsinglass. Thepreisenature
of thetransition remainsontroversial;forreviewssee
Refs. [43℄ and[56℄.
VII Catalysis Models
Another large lass of nonequilibrium lattie models
arisesinthestudyofheterogeneousatalysis,typially
on ametalli surfae. Oneof therst suh modelsto
bestudiedindetailwasintroduedbyZi,Gulariand
Barshad(ZGB),todesribethereationCO+1/2O
2
! CO on aplatinumsurfae [57℄. These models
typ-ially exhibit transitions to an absorbingstate, whih
(whenontinuous)fallin thediretedperolationlass
[58℄;theyarereviewedinhapter5ofRef. [43℄.
TheZGBmodelexhibitstwophasetransitions,one
ontinuous, the other disontinuous. Under asuitable
point, whih appears to belong to the Ising lass. In
theZGBmodel,theatalytisurfaeisrepresentedby
atwodimensionallattie. Thetransition ratesinvolve
asingleparameter,Y: theprobabilitythat amoleule
arrivingatthesurfaeisCO.Thismoleuleneedsonlya
singlevaantsitetoadsorb,asindiatedby
experimen-talstudies;O
2
requiresapairofvaantsites. Aftereah
adsorptionevent,thesurfaeisimmediatelylearedof
anyCO-Onearest-neighbor(NN)pairs,makingthe
re-ationrate for CO
2
formation, in eet, innite. The
adsorptionandreationeventsomprisingthe
dynam-is go as follows. First hoose the adsorbing speies
|COwith probabilityY, O
2
with probability1 Y
| and a lattie site x (or, in the ase of O
2 , a NN
pair,(x,y)), at random. Ifx is oupied (forO
2 , if x
and/oryareoupied),theadsorptionattemptfails. If
thenewly-adsorbedmoleuleisCO,determinethe set
O(x)of NNs of x harboring an Oatom. If this set is
empty, the newly-arrived COremains at x; otherwise
itreats,vaatingxandoneofthesitesinO(x),
(ho-sen at random if O(x) ontains more than one site).
If thenewly-adsorbed moleuleis O
2
, onstrutC(x),
theset of neighbors of x harboring CO, and similarly
C(y). TheOatomatxremains(reats)ifC(x)isempty
(nonempty),andsimilarlyfortheatomaty.
At a threshold value of Y, y
2
(' 0:5256 on the
square lattie), the ZGB model is said to exhibit
\CO-poisoning." That is,the stationarystatehanges
abruptlyfromanativeone (Y <y
2
),withontinuous
produtionofCO
2
, toanabsorbingstatewithallsites
oupied by CO. (With all sites bloked, there is no
way for O
2
to adsorb.) The transition is sharply
dis-ontinuous. Experiments on surfae-atalyzed CO +
1/2O
2
!COreationsdoshowadisontinuous
tran-sitionbetweenstatesofhigh and lowreativityasthe
partialpressurep
CO
(analogousto Y inthemodel),is
inreased[59℄. With inreasingtemperature,the
tran-sition softens, and at a ertain temperature beomes
ontinuous, about whih the reation rate beomes a
smoothfuntionofp
CO
. Thisappearstobetheresult
ofthermally-ativated,nonreativedesorptionofCO.
A similar sequene of alterations is observed if we
inludenonreativedesorptionofCOataertainrate,
k,in theZGBmodel. With COdesorptionthere isno
longeraCO-poisonedstate,butforsmallkthe
dison-tinuous transitionbetweenlowandhighCO overages
persists. Abovearitial desorptionrate k
'0:0406,
the overages (and the CO
2
prodution rate) vary
smoothly with Y [60, 61, 62℄. Simulation results
in-diate that at the ritial point of the COtransition,
the ritial exponent = 1, and the redued fourth
two-VIII Hysteresis in the Ising Model
For our nal example we ome full irle to the Ising
model,foredoutofequilibrium,thistime,notthrough
abiasoronitingdynamis,butbyatime-dependent
externaleldh(t). Ofprinipalinterestisaperiodially
varying eld, for example, h(t)sinusoidal or asquare
wave,with
hhi 1
T Z
T
0
h(t)dt=0; (8)
where T is the period of osillation. Well below the
ritial temperature, and at suÆiently low
frequen-ies, we expet to observe a hysteresis loop in the
magnetization-eld plane. As we raise the frequeny,
the systemwill at somepointbeunable to followthe
rapidvariationsoftheeld,andthemagnetizationm(t)
will remain lose to one of its stationary values, i.e.,
+m
0 or m
0
. Thatis, forhigh frequeniesweexpet
Q 1
T Z
T
0
m(t)dt6=0 (9)
whereasatlowfrequeniesQshould bezero.
Forasinusoidaleld,thetime-dependentmean-eld
equation desribing this system predits aontinuous
transition between a dynamially disordered (Q = 0)
and a dynamially ordered (Q 6= 0) state at a
riti-al frequeny, whih depends onthe temperature and
h
0
= max[h(t)℄ [63℄. The transition was onrmed in
various Monte Carlosimulations,andmayberelevant
toexperimentsonthinmagnetilms[64,65℄.
Rikvold and oworkers suggested that the
transi-tionbetweendynamiallyorderedanddisorderedstates
ours when the period T beomes omparable to
the metastable lifetime (h
0
;T) of the state with the
\wrong" signof themagnetization (in astatield of
magnitudeh
0
),and presentedextensivesimulation
re-sultstosupportthisproposal[66℄. Theseauthorsmade
adetailednumerialstudyoftheritialbehaviorofQ
intwodimensions;theirresultsforexponentratiosand
the fourth umulant are onsistent with Ising values.
While the very general symmetry arguments outlined
aboveagainfavorsuhaonlusion,ndingapathfrom
themirosopidynamisofthisnonstationaryproess
to anequation oftheformof Eq. (7)seemsa
partiu-larlyhallengingtheoretialtask.
VI Summary
Ihavereviewedanumberofexamplesinwhihritial
behaviorintheIsinguniversalitylassappears,despite
the preseneof long-rangeinterations, lakof a
tem-perature sale, or lak of equilibrium. The
Ornstein-Zernike approah that omes losest to reproduing
Ising-like behaviorwas also reviewed. A general
on-of the dynamis - are essentialto determining ritial
behavior; thequestion ofequilibrium isof minimal, if
any,signiane. Ontheotherhand,wehaveseenthat
the eetive, oarse-grained dynamis an look quite
dierent from the mirosopi interations. In many
ases there remains aonsiderable gap between these
twolevelsofdesription.
Aknowledgements
It is a pleasure to thank George Stell and T^ania
Tomeforollaborationsthatled tosomeoftheresults
desribed in this work. This work was supported by
CNPq, FapemigandMCT.
Referenes
[1℄ L. S.Ornstein andF.Zernike,Pro.Aad. Si.
Ams-terdam17,793(1914).
[2℄ J. P.Hansen and I. R. MDonald, Theory of Simple
Liquids,2nded.AademiPress,London,1986.
[3℄ R.Balesu,EquilibriumandNonequilibriumStatistial
Mehanis,Wiley,NewYork,1975.
[4℄ Note that (r) plays a role analogous to a two-point
vertexfuntionineldtheory.
[5℄ J.S.HyeandG.Stell,J.Chem.Phys.67,439(1977);
Mole. Phys. 52, 1057, 1071 (1984); Int. J.
Thermo-phys.6,561(1985).
[6℄ R. Dikman and G. Stell, Phys. Rev. Lett. 77, 996
(1996).
[7℄ D. Pini, G..Stell, andR.Dikman, Phys.Rev.E 57,
2862(1998).
[8℄ C.F.Baillieetal.,Phys.Rev.B45,10438(1992).
[9℄ M. F. Sykes et al., J. Phys. A: Math. Gen. 5, 640
(1972).
[10℄ A.J.LiuandM. E.Fisher,PhysiaA156,35(1989).
[11℄ D. Pini,G.Stell,andN.Wilding,Mol.Phys.95,483
(1998).
[12℄ E. Kierlik, M. L. Rosinberg, and G. Tarjus, J. Stat.
Phys.89,215(1997).
[13℄ R.R.SinghandK.S.Pitzer,J.Chem.Phys.92,6775
(1990).
[14℄ M. L. Japas and J. M. H. Levelt Sengers, J. Phys.
Chem.94,5361 (1990).
[15℄ T.Narayanand K.S.Pitzer,J.Phys.Chem98,9170
(1994);Phys.Rev.Lett.73,3002 (1994).
[16℄ P.P.DebyeandF.Hukel,Phys.Z.24,185(1923).
[17℄ G.Stell,K.C.Wu,andB.Larsen,Phys.Rev.Lett.37,
1369(1976).
[18℄ J. M. Caillol, D. Levesque,and J.J. Weis, J. Chem.
Phys.107,1565(1997).
[19℄ J.P.Valleau,andG.Torrie,J.Chem.Phys.108,5169
(1998).
[21℄ B.Hafskj ldandG.Stell,inStudiesinStatistial
Me-hanis Vol. III, E. W. Montroll and J. L. Lebowitz,
eds.,North-Holland,Amsterdam,1982.
[22℄ G.Stell,Phys.Rev.A45,7628 (1992).
[23℄ M.E.Fisher,J.Stat.Phys.75,1(1994).
[24℄ Y.LevinandM.E.Fisher,PhysiaA225,164(1996).
[25℄ A. Ciah and G.Stell, J. Mole. Liquids, to appear.
e-print: ond-mat/0007286.
[26℄ R.DikmanandG.Stell,inSimulationandTheoryof
Eletrostati Interations inSolution,L. R.Prattand
G. Hummer, eds., AIP Conferene Proeedings 492,
Melville,NY,1999.
[27℄ A. Z. Panagiotopoulos and S.K. Kumar, Phys.Rev.
Lett.83,2981(1999).
[28℄ J. Frolih, R. Israel, E. Lieb, and B. Simon, J.Stat.
Phys.22,296(1980).
[29℄ B.WidomandJ.S.Rowlinson,J.Chem.Phys.52,1670
(1970).
[30℄ J. S. Rowlinson and B.Widom, Moleular Theory of
Capillarity,ClarendonPress,Oxford,1982.
[31℄ E. Helfand and F. H. Stillinger, J. Chem. Phys. 49,
1232 (1968).
[32℄ R. Dikmanand G. Stell, J. Chem. Phys.102, 8674
(1995).
[33℄ M.E.Fisher,Phys.Rev.176,257(1968).
[34℄ S.-N.LaiandM.E.Fisher,J.Chem.Phys.103,8144
(1995).
[35℄ R. Sun,H.Gould,J.Mahta,and L. Chayes,e-print:
ond-mat/0003516.
[36℄ D.S.GauntandM.E.Fisher,J.Chem.Phys.43,2840
(1965).
[37℄ D. S.Gaunt,J.Chem.Phys.46,3237(1967).
[38℄ L. K.Runnelsand L. L. Combs, J. Chem. Phys.45,
2482 (1966).
[39℄ W.Kinzel,Z.Phys.B58,229(1985).
[40℄ SeeBraz.J.Phys.30,no.1(Marh,2000)fora
olle-tionofartilesonthissubjet.
[41℄ R. Dikman, in Nonequilibrium Statistial Mehanis
in One DimensionV. Privman, ed., Cambridge
Uni-versityPress,Cambridge1996.
[42℄ G.GrinsteinandM.A.Mu~noz,inFourthGranada
Le-turesinComputationalPhysis,Ed.P.GarridoandJ.
Marro, LetureNotes inPhysis, 493, 223
Springer-Verlag,Berlin,1997.
[43℄ J.MarroandR.Dikman,NonequilibriumPhase
Tran-sitions in Lattie Models CambridgeUniversityPress,
Cambridge,1999.
[44℄ T.M. Liggett,Interating Partile Systems,
Springer-Verlag,(NewYork,1985).
[45℄ J.W.Essam,J.Phys.A22,4927(1989).
[46℄ R.Dikmanand A. Yu.Tretyakov,Phys.Rev.E 52,
3218(1995).
[47℄ M.J.deOliveira,J.Stat.Phys.66,273(1992).
[48℄ M.J.deOliveira,J.F.F.MendesandM. A.Santos,
J.Phys.A26,2317 (1993).
[49℄ M. A. Santosand S.Teixeira, J.Stat. Phys.78, 963
(1995).
[50℄ T.TomeandJ.R.DrugowihdeFelio,Phys.Rev.E
53,3976(1996).
[51℄ P.L.Garrido,A.Labarta,andJ.Marro,J.Stat.Phys.
49,551(1987).
[52℄ T.TomeandM.J.deOliveira,Phys.Rev.E58,4242
(1998).
[53℄ G.Grinstein,C. Jayaprakash,and Y. He, Phys.Rev.
Lett.55,2527(1985).
[54℄ A. Brunstein and T. Tome, Phys. Rev. E 60, 3666
(1999).
[55℄ T.Tome,Braz.J.Phys.30,152(2000).
[56℄ B.ShmittmannandR.K.P.Zia,Statistial
Mehan-isofDrivenDiusiveSystems,vol.17ofPhase
Tran-sitions and Critial Phenomena, C. Domb and J. L.
Lebowits.eds.,AademiPress,NewYork,1995.
[57℄ R.M.Zi,E.Gulari,andY.Barshad,Phys.Rev.Lett.
56,2553(1986).
[58℄ G.Grinstein,D.-W.Lai,andD.A.Browne,Phys.Rev.
A40,4820(1989).
[59℄ M.Ehsasietal.,J.Chem.Phys.91,4949(1989).
[60℄ B.J.Brosilowand R.M.Zi, Phys.Rev.A46,4534
(1992).
[61℄ J.W.Evans,J.Chem.Phys.97,572(1992).
[62℄ T.TomeandR.Dikman,Phys.Rev.E47,948(1993).
[63℄ T.TomeandM.J.deOliveira,Phys.Rev.A41,4251
(1990).
[64℄ M. Aharyya and B. K.Chakrabarti, in Annual
Re-viewsof ComputationalPhysis,v.1,D.Stauer,ed.,
WorldSienti,Singapore,1994.
[65℄ B.K.ChakrabartiandM.Aharyya,Rev.Mod.Phys.
71,847(1999).
[66℄ P.A. Rikvold etal., inComputer Simulation Studies
in Condensed-Matter Physis XII, D. P. Landau, S.
P. Lewis, and H.-B. Shuttler, eds., Springer-Verlag,