Lat. Am. j. solids struct. vol.11 número14

 

Abstract

Wave propagation in heat conducting thermo elastic plate of ellip-tical cross-section is studied using the Fourier expansion colloca-tion method based on Suhubi’s generalized theory. The equacolloca-tions of motion based on two-dimensional theory of elasticity is applied under the plane strain assumption of generalized thermo elastic plate of elliptical cross-sections composed of homogeneous iso-tropic material. The frequency equations are obtained by using the boundary conditions along outer and inner surface of elliptical cross-sectional plate using Fourier expansion collocation method. The computed non-dimensional frequency, velocity and quality factor are plotted in dispersion curves for longitudinal and flexural (symmetric and antisymmetric) modes of vibrations.

Keywords

Wave propagation in plate; vibration of thermal plate; Fourier collocation method; generalized thermo elastic plate; thermal re-laxation times.

Modeling and analysis of waves in a heat conducting

thermo-elastic plate of elliptical shape

1 INTRODUCTION

The plates of elliptical cross-sections are frequently used as structural components and their vi-bration characteristics are important for practical design. The propagation of waves in thermoe-lastic material has many applications in various fields of science and technology, namely, atomic physics, industrial engineering, thermal power plants, submarine structures, pressure vessel, aero-space, chemical pipes, and metallurgy. The importance of thermal stresses in causing structural damages and changes in functioning of the structure is well recognized whenever thermal stress environments are involved.

A method, for solving wave propagation in doubly connected arbitrary and polygonal cross-sectional plates and to find out the phase velocities in different modes of vibrations namely longi-tudinal, torsional and flexural, by constructing frequency equations was devised by Nagaya (1981a; 981b; 1983a; 1983b; 1983c). He formulated the Fourier expansion collocation method for

R. Selvamania P. Ponnusamyb

a_{Department of Mathematics,}

Karunya University, Coimbatore-641 114, Tamil Nadu, India.

b_{Departments of Mathematics,}

Govt Arts College, Coimbatore, 641 018,

Tamil Nadu, India.

a_{Corresponding author:} selvam1729@gmail.com

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

this purpose, the same method is adopted in this problem. The generalized theory of thermo elas-ticity was developed by Lord and Shulman (1967) involving one relaxation time for isotropic ho-mogeneous media, which is called the first generalization to the coupled theory of elasticity. These equations determine the finite speeds of propagation of heat and displacement distribu-tions, the corresponding equations for an isotropic case were obtained by Dhaliwal and Sherief (1980). The second generalization to the coupled theory of elasticity is what is known as the theo-ry of thermo elasticity, with two relaxation times or the theotheo-ry of temperature-dependent ther-moelectricity. A generalization of this inequality was proposed by Green and Laws (1972). Green and Lindsay (1972) obtained an explicit version of the constitutive equations. This theory con-tains two constants that act as relaxation times and modify not only the heat equations, but also all the equations of the coupled theory. The classical Fourier’s law of heat conduction is not vio-lated if the medium under consideration has a center of symmetry. Erbay and Suhubi (1986) studied the longitudinal wave propagation in a generalized thermoplastic infinite cylinder and obtained the dispersion relation for a constant surface temperature of the cylinder.

Sharma and Pathania (2005) investigated the generalized wave propagation in circumferential curved plates. Asymptotic of wave motion in transversely isotropic plates was analyzed by Shar-ma and KuShar-mar (2012). Tso and Hansen (1995) have studied the wave propagation through cylin-der/plate junctions. Heyliger and Ramirez (2000) analyzed the free vibration characteristics of laminated circular piezoelectric plates and disc by using a discrete-layer model of the weak form of the equations of periodic motion. Thermal deflection of an inverse thermo elastic problem in a thin isotropic circular plate was presented by Gaikward and Deshmukh (2005). Verma and Hasebe (2001) investigated the wave propagation in plates of general anisotropic media in gener-alized thermo elasticity. Later, Verma (2002) has presented the propagation of waves in layered anisotropic media in generalized thermo elasticity in an arbitrary layered plate. The free vibration of non-homogeneous transversely isotropic magneto-electro-elastic plates was studied by Chen et al. [19]. Kumar and Partap (2007) presented the free vibration of microstretch thermoelastic plate with one relaxation time. Ponnusamy and Selvamani (2012) have studied the dispersion analysis of generalized magneto-thermo elastic waves in a transversely isotropic cylindrical panel using the wave propagation approach. Later, Selvamani (2012) performed mathematical modeling and analysis for damping of generalized thermoelastic waves in a homogeneous isotropic plate.

In this paper, the in-plane vibration of heat conducting thermo elastic elliptical cross-sectional plate of homogeneous isotropic material is studied. The solutions to the equations of motion for an isotropic medium is obtained by using the two dimensional theory of elasticity. To satisfy the boundary conditions, the Fourier expansion collocation method is performed to the equations of the boundary conditions and the frequency equations are obtained for longitudinal and flexural (symmetric and antisymmetric) modes of vibrations. The computed non-dimensional frequency, velocity and quality factor are plotted in dispersion curves for longitudinal and flexural (symmet-ric and antisymmet(symmet-ric) modes of vibrations.

2 FORMULATION OF THE PROBLEM

displace-Latin American Journal of Solids and Structures 11 (2014) 2589-2606

ments and stresses are defined in polar coordinates r and q in an arbitrary point inside the plate

and denote the displacements u_r in the direction of r and u_q in the tangential direction q.

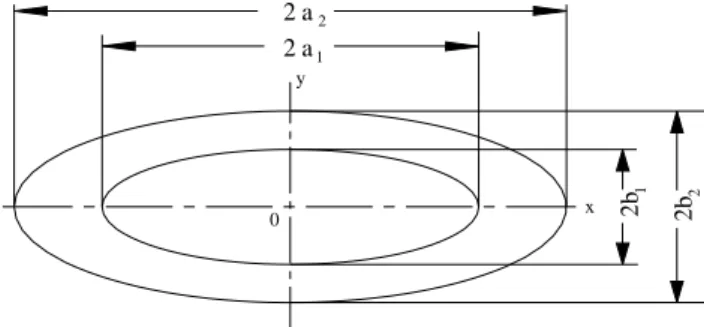
Figure 1: Geometry of ring-shaped elliptical plate.

The two dimensional stress equations of motion, and strain –displacement relations and heat con-duction equation in the absence of body force for a linearly elastic medium are

(

)

(

)

1 1

, , ,

1 1

, , ,

1 2 1

, , , , , 0 , , ,

2

( )

rr r r rr r tt

r r r tt

rr r t tt r rt r t t

r r u

r r u

K c T r T r T c T T T u r u u

q q qq

q qq q q q

n qq n q q

s s s s r

s s s r

r r rt b

-

--

-- -

-+ + - =

+ + =

é ù

+ + = + + _êë + + _úû

(1)

and

(

)

(

)

(

)

(

,,

)

2 2 2

rr rr rr t

rr t

r r

e e e T T

e e e T T

e

qq

qq qq qq

q q

s l m b h

s l m b h

s m

= + + - +

= + + - +

=

(2)

where s_rr, , s_qq s_rq are the stress components, e_rr, , e_qq e_rq are the strain components, T is the

temperature change about the equilibrium temperature T₀, r is the mass density, c_v is the

spe-cific heat capacity, b is a coupling factor that couples the heat conduction and elastic field equa-tions, K is the thermal conductivity , h t, is the thermal relaxation times, t is the time, l and

m are Lame’ constants.

The strain e_ij related to the displacements are given by

,

rr r r

e =u , 1

(

)

,

r

e_qq =r- u +u_{q q} , e_rq =u_q,r -r-1

(

u_q -u_r,q

)

(3)

in which u_r and u_q are the displacement components along radial and circumferential directions,

respectively. The comma in the subscripts denotes the partial differentiation with respect to the variables.

Substituting Eqs. (3) and (2) in Eq. (1), the following displacement equations of motions are ob-tained

0 x

y

2 a1 2 a2

2b

1

2b

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

1 2 2 1 2

, , , , , , , ,

1 2 2 2 1

, , , , , , _, ,

1 2 1

, , , , , 0 ,

2 3

2 3

(

r rr r r r r r t _r r tt

rr r r r r t tt

rr r t tt r tr

u r u r u r u r u r u T T u

u r u r u r u r u r u T T u

K c T r T r T c T T T u r

qq q q q q

q q q q qq q q _q q

n qq n

l m m l m l m b h r

m l m l m l m b h r

r r rt b

- - - -

-- - - -

-- -

-+ + - + + + + + - + =

+ - + + + + + + - - + =

+ + = + + _ëéê + ur t_, +uq q_,t )ùú_û

(4)

3 SOLUTIONS OF THE PROBLEM

Eq. (4) is a coupled partial differential equation with two displacements and heat conduction components. To uncouple Eq.(4), we follow Mirsky (1964) by assuming the vibration and dis-placements along the axial direction z equal to zero. Hence assuming the solutions of Eq. (4) in the form

(

)

(

)

(

)

(

)

(

)

(

)

1 1

, ,

, ,

0

1 1

, ,

, ,

0

2

0

( , , , ) ( , , , )

( , , , ) 2

i t n n r

r n n r n

n

i t n r n

n n n r n

i t n n n n

u r z t r r e

u r z t r r e

T r z t a T T e

w q q

w q

q q

w

q e f y f y

q e f y f y

q l m b e

¥

-

-= ¥

-

-=

¥ =

é ù

= _êë + + + _úû

é ù

= _êë - + - _úû

= + +

å

å

å

(5)

where e_n =1 2 for n=0, e_n =1 for n ³1, i= -1, w is the frequency, f_n

( )

r,q ,

( )

,

n r

y q , T_n

( )

r,q , f_n

( )

r,q ,y_n

( )

r,q and Tn

( )

r,q are the displacement potentials.

Introducing the dimensionless quantities such as T_a =t m r a, x =r a, c₁2 =

(

l+2m r

)

,

1

c a K

a¢ = , 2 2 2 2

1

a c

w

W = , 2

(

2

)

1 T a0 c c Kv 1

e = b r , 2

(

)

2 c1 c Kn

e = t , and e₃ =c₁h a and using

Eq. (5) in Eq. (4), we obtain

(

_{A +  +}4 _B 2 _C

)

_{f=0 (6)}

where

1

A= , B = W2

(

1+e e_{1 3}

)

+e₂ - Wi

(

a' +e₁

)

, c = W2

(

e₂ -ia'W

)

(7)

and

(

)

(

2 ₂ 2 2

)

₀

n

l V y

 + + W - = (8)

where _{ º ¶ ¶}2 2 _x2 _+x-1_{¶ ¶ +x x}-2_{¶ ¶}2 _q2

The parameters defined in Eq. (7) namely, e₁ couples the equations corresponding to the elas-tic wave propagation and the heat conduction which is called the coupling factor; the coefficient

2

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

(

)

(

)

2

1

cos

n in n i in n i i

A J ax B Y ax n

f a a q

=

é ù

=

å

_ë + _û (9.a)

(

)

(

)

2

1

cos

n i in n i in n i i

T d A J aax B Y aax nq

=

é ù

=

å

_ë + _û (9.b)

and the solution for the antisymmetric mode fn is obtained by replacing cosnq by sinnq in Eqs.

(9a) and (9b), we get

(

)

(

)

2

1

sin

in in

n n i n i

i

A J ax B Y ax n

f a a q

=

é ù

=

å

_êë + _úû (10.a)

(

)

(

)

2

1

sin

n _i in _{n i} in _{n i} i

T d A J aax B J aax nq

=

é ù

=

å

_êë + _úû (10.b)

where Jn is the Bessel function of first kind of order n and Yn is the Bessel function of second

kind of order n. Solving Eq. (8), we obtain

(

)

(

)

3 3 3 3 sin

n A Jn n ax B Yn n ax n

y = é_ë a + a ù_û q (11.a)

for symmetric mode, and for the antisymmetric mode y_n is obtained from Eq. (11a) by replacing

sinnq by cosnq.

(

)

(

)

3n ₃ 3n ₃ cos

n A Jn ax B Jn ax n

y = é_ê a + a ù_ú q

ë û (11.b)

where

(

)

2

(

)

2 2

3a 2

a = +l W -V . If

(

a_ia

)

2 <0(i=1, 2, 3), then the Bessel functions J_n and Y_n are replaced by the modified Bessel function In and Kn respectively .

4 BOUNDARY CONDITIONS AND FREQUENCY EQUATIONS

In this problem, the vibration of thick arbitrary cross-sectional plate is considered. Since the boundary is irregular in shape, it is difficult to satisfy the boundary conditions along both outer and inner surface of the plate directly. Hence, the Fourier expansion collocation method is applied to satisfy the boundary conditions. For the plate, the normal stress '

xx

s , shearing stress '

xy

s and thermal field _T' _{along the inner surface of the plate is equal to zero. Similarly, normal stress}

xx

s , shearing stress s_xy and thermal field T along the outer surface of the plate is equal to zero. Thus, the boundary conditions along the outer boundary of the plate are

(

_xx

)

( )

_xy

( )

0

i i T i

s = s = = (12)

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

( ) ( ) ( )

' ' ' ₀

xx _i xy _i T _i

s = s = = (13)

where x is the coordinate normal to the boundary and y is the coordinate tangential to the

boundary, s_xx, '

xx

s are the normal stresses, s_xy, '

xy

s are the shearing stresses, T , _T' _{are the} thermal fields and

( )

_i is the value at the i-th segment of the outer and inner boundary re-spectively. Since the cross-section of the plate is irregular, it is difficult to find the transformed expression of the stresses in the thick arbitrary cross-sectional plates because the coordinate x

and y are vary with the angleq. Therefore, the inner and outer boundary of the thick arbitrary cross-sections is divided into small segments such that the variations of the stresses are assumed to be constant. Assuming the angle g_i, between the normal to the segment and the reference axis to be constant, the transformed expressions for the stresses are followed by Nagaya (1983b) as

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

)

(

(

)

)

(

)

' 1 2 1 2

, , , ,

1

, , ,

' 1 1

, , , ,

2 [ cos sin

0.5 sin 2 ]

[ sin 2 sin 2 ]

xx r r r r r i r i

r r i t

xy r r r i _r r i

u r u u u r u u

r u u u T T

u r u u r u u u

q q q q

q q q

q q q q q

s l m q g q g

q g b h

s m q g q g

- -- -= + + + - + + -+ - - - - + = - + - + - + (14)

Substituting Eqs. (9)-(11)in Eqs. (12) and (13), the boundary conditions are transformed as fol-lows:

( )

( )

( )

' ' ' ' ' ' 0 0 0 a a a i T xx xx i i i T xy xy i i i T t t i i

S S e

S S e

S S e

W W W

é æ_{ç ö÷} ù

ê +ç_ç ÷_÷ ú =

ê è ø ú

ë û

é æ_{ç ö÷} ù

ê +ç_ç ÷_÷ ú =

ê è ø ú

ë û

é æ_{ç ö÷} ù

ê +ç_ç ÷_÷ ú =

ê è ø ú

ë û

(15)

for the inner surface and

( )

( )

( )

( )

( )

( )

0 0 0 a a a i T xx xx i _i

i T xy xy i i

i T t t i _i

S S e

S S e

S S e

W W W

é ₊ ù ₌

ê ú

ë û

é ₊ ù ₌

ê ú

ë û

é ₊ ù ₌

ê ú

ë û

(16)

for the outer surface, where

1 2 3 4 1 2 3 4 5 6

'

0 ₁₀ 0 ₁₀ 0 ₂₀ 0 _{20 1 1 2 2 3 3}

1

1 2 3 4 1 2 3 4

'

0 10 0 10 0 20 0 20 1 1 2 2

0.5

0.5

n n n n n n

xx n n n n n n

n

n n n n n

xy n n n n

S e A e B e A e B e A e B e A e B e A e B

S f A f B f A f B f A f B f A f B f

¥

=

æ ö_÷ æ ö_÷

ç ç

= _çç + + + ÷ +_÷÷ ç_ç + + + + + _÷÷_÷

è ø è ø

æ _ö÷

ç

= ç_ç + + + ÷ +_÷÷ + + + +

è ø

å

         

        5 6

3 3

1

1 2 3 2 1 2 3 4 5 6

'

0 10 0 10 0 20 0 20 1 1 2 2 3 3

1 0.5

n

n n

n

n n n n n n

t n n n n n n

n

A f B

S g A g A g A g A g A g B g A g B g A g B

¥

= ¥

=

æ _ö÷

ç _{+ ÷}

ç _÷

ç ÷

è ø

æ ö_÷ æ ö_÷

ç ç

= _çç + + + ÷ +_÷÷ ç_ç + + + + + _÷÷_÷

è ø è ø

å

å



         

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

(

)

(

)

(

)

(

)

(

)

1 2 3 4 1 2 3 4 5 6

0 10 0 10 0 20 0 20 1 1 2 2 3 3

1

1 2 3 4 1 2 3 4 5 6

0 10 0 10 0 20 0 20 1 1 2 2 3 3

1

1 2 3 4 1

0 10 0 10 0 20 0 20 0.5

0.5

0.5

xx n n n n n n n n n n n n

n

xy n n n n n n n n n n n n

n

t n

S e A e B e A e B e A e B e A e B e A e B

S f A f B f A f B f A f B f A f B f A f B

S g A g B g A g B g A

¥ = ¥ = = + + + + + + + + + = + + + + + + + + + = + + + +

å

å

(

2 3 4 5 6

)

1 1 2 2 3 3

1

n n n n n n n n n n n

n

g B g A g B g A g B

¥

=

+ + + + +

å

(17.b)

3 4 1 2 3 4 5 6

'

0 20 0 20 1 1 2 2 3 3

1

3 4 1 2 3 4 5 6

'

20 20 1 1 2 2 3 3

0 0

0.5

0.5

xx n n n n n n n n n n n n

n

xy _n n _n n _n n _n n _n n _n n

n

S e A e B e A e B e A e B e A e B

S f A f B f A f B f A f B f A f B

¥ =

æ ö_÷ æ ö_÷

ç _÷ ç _÷

ç ç

= _çç + _÷÷÷+ _çç + + + + + _÷÷÷

è ø è ø

æ ö_÷ æ ö_÷

ç _÷ ç _÷

ç ç

= _çç + _÷÷÷+ _çç + + + + + _÷÷÷

è ø è ø

å

       

       

1

3 4 1 2 3 4 5 6

'

20 20 1 1 2 2 3 3

0 0

1

0.5

t _n n _n n _n n _n n _n n _n n

n

S g A g B g A g B g A g B g A g B

¥ = ¥

=

æ ö_÷ æ ö_÷

ç _÷ ç _÷

ç ç

= _ç + _÷÷+ _ç + + + + + _÷÷

÷ ÷

ç ç

è ø è ø

å

å

       

(18.a)

3 4 1 2 3 4 5 6

0 20 0 20 1 1 2 2 3 3

1

3 4 1 2 3 4 5 6

20 20 1 1 2 2 3 3

0 0 1 3 4 20 20 0 0 0.5 0.5 0.5

xx n n n n n n n n n n n n

n

xy _n n _n n _n n _n n _n n _n n

n

t

S e A e B e A e B e A e B e A e B

S f A f B f A f B f A f B f A f B

S g A g B

¥ = ¥

=

æ ö_÷ æ ö_÷

ç ç

= _çèç + ÷_÷ø+ ç_çè + + + + + ÷_÷ø

æ ö_÷ æ ö_÷

ç ç

= _çèç + ÷_÷ø+ ç_çè + + + + + ÷_÷ø

æ ö

ç

= _ççè +

ø

å

å

1 2 3 4 5 6

1 1 2 2 3 3

1

n n n n n n

n n n n n n

n

g A g B g A g B g A g B

¥ =

æ ö

÷₊ ç _{+ + + + +} ÷

÷ ç ÷

÷

å

_çè ÷_ø

(18.b)

The coefficients for ein -gi_n are given in the Appendix A.

Performing the Fourier series expansion to Eqs. (12) and (13) along the boundary, the bound-ary conditions along the inner and outer surfaces are expanded in the form of double Fourier se-ries. When a plate is symmetric about more than one axis, the boundary conditions, in the case of symmetric mode can be written in the form of a matrix as given below:

               

               

          

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

00 00 00 00 01 0 01 0 01 0 01 0 01 0 01 0

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 0 0 1 1 1 1 1 1

1 2 3 4 1 1 2 2 3 3

10 10 10 10 11 1 11 1 11 1

N N N N N N

N N N N N NN N NN N NN N NN N NN N NN

N N N

E E E E E E E E E E E E E E E E

E E E E E E E E E E E E E E E E

F F F F F F F F F F F

                                                                    

4 4 5 5 6 6

11 1 11 1 11 1

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 0 0 1 1 1 1 1 1

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

00 00 00 00 01 0 01 0 01 0 01 0 01 0 01 0

N N N

N N N N N NN N NN N NN N NN N NN N NN

N N N N N N

N

F F F F F

F F F F F F F F F F F F F F F F

G G G G G G G G G G G G G G G G

G                                                              

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 0 0 1 1 1 1 1 1

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

00 00 00 00 10 0 10 0 10 0 10 0 10 0 10 0

1 2 3 4 1 1 2 2 3 3

0 0 0 0 1 1 1 1

N N N N NN N NN N NN N NN N NN N NN

N N N N N N

N N N N N NN N NN N NN N

G G G G G G G G G G G G G G G

E E E E E E E E E E E E E E E E

E E E E E E E E E E E

     

     

               

   4 4 5 5 6 6

1

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

10 10 10 10 11 1 11 1 11 1 11 1 11 1 11 1

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 0 0 1 1 1 1 1 1

1 2 3 4 1

00 00 00 00 00

NN NN NN N NN

N N N N N N

N N N N N NN N NN N NN N NN N NN N NN

E E E E E

F F F F F F F F F F F F F F F F

F F F F F F F F F F F F F F F F

G G G G G

  

     

               

     

1 2 2 3 3 4 4 5 5 6 6

0 00 0 00 0 00 0 00 0 00 0

1 2 3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 0 0 1 1 1 1 1 1 1 1

N N N N N N

N N N N N NN N NN N NN N N N N N NN

G G G G G G G G G G G

G G G G G G G G G G G G G G G G

Latin American Journal of Solids and Structures 11 (2014) 2589-2606 where 

₍

₎

_{( )}

 

₍

₎

_{( )}

 

₍

₎

_{( )}

 1 1 1 1 1 1

2 , cos

2 , sin

2 , cos

i i i i i i I i i

mn _{n n} i

i I i

i

mn _{n n} i

i I i

i

mn _{n n} i

i

E e R m d

F f R m d

G g R m d

q q q q q q

e p q q q

e p q q q

e p q q q

-= = = = = =

å ò

å ò

å ò

(20.a)

(

)

(

)

(

)

(

)

(

)

(

)

1 1 1 1 1 1

2 , cos

2 , sin

2 , cos

i i i i i i I i i

mn n n i

i I

i i

mn n n i

i I

i i

mn n n i

i

E e R m d

F f R m d

G g R m d

q q q q q q

e p q q q

e p q q q

e p q q q

-= = = = = =

å ò

å ò

å ò

(20.b)

Similarly, the matrix for the antisymmetric mode is obtained as

             

             

             

3 4 1 1 2 2 3 3 4 4 5 5 6 6

10 10 11 1 11 1 11 1 11 1 11 1 11 1

3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 1 1 1 1 1 1

3 4 1 1 2 2 3 3 4 4 5 5 6 6

00 00 01 0 01 0 01 0 01 0 01 0 01 0

N N N N N N

N N N NN N NN N NN N NN N NN N NN

N N N N N N

E E E E E E E E E E E E E E

E E E E E E E E E E E E E E

F F F F F F F F F F F F F F

                                                                      

3 3 1 1 2 2 3 3 4 4 5 5 6 6

0 0 1 1 1 1 1 1

3 4 1 1 2 2 3 3 4 4 5 5 6 6

10 10 11 1 11 1 11 1 11 1 11 1 11 1

3 4 1 1 2 2 3 3 4 4 5

0 0 1 1 1 1 1

N N N NN N NN N NN N NN N NN N NN

N N N N N N

N N N NN N NN N NN N NN N

F F F F F F F F F F F F F F

G G G G G G G G G G G G G G

G G G G G G G G G G G

             

     

     

             

    5 61 6

3 4 1 1 2 2 3 3 4 4 5 5 6 6

10 10 11 1 11 1 11 1 11 1 11 1 11 1

3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 0 1 1 1 1 1 1

3 4 1 1 2 2 3 3 4 4 5

00 00 01 0 01 0 01 0 01 0 01

NN N NN

N N N N N N

N N N NN N NN N NN N NN N NN N NN

N N N N

G G G

E E E E E E E E E E E E E E

E E E E E E E E E E E E E E

F F F F F F F F F F F

 

     

             

     

    50 601 60

3 4 1 1 2 2 3 3 4 4 5 5 6 6

0 10 1 1 1 1 1 1

3 4 1 1 2 2 3 3 4 4 5 5 6 6

10 10 11 1 11 1 11 1 11 1 11 1 11 11

3 4 1 1 2 2 3

0 0 1 1 1

N N

N N NN N NN N NN N NN N NN N NN

N N N N N

N N N NN N NN N NN

F F F

F F F F F F F F F F F F F F

G G G G G G G G G G G G G G

G G G G G G G G

                                             30 30 11 1 11 1 21 2 21 2 31 3 31

3 4 4 5 5 6 6 3

1 1 1

N N N N N N

N NN N NN N NN

A B A A B B A A B B A A B B

G G G G G G

é ù

ê _{ú é}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê _{ú ê}

ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú ê ú

ê ú_ë

Latin American Journal of Solids and Structures 11 (2014) 2589-2606 

₍

₎

_{( )}

 

₍

₎

_{( )}

 

₍

₎

_{( )}

 1 1 1 1 1 1

2 , sin

2 , cos

2 , sin

i i i i i i I j _j

mn _n n i

i I

j _j

mn _{n n} i

i I

j _j

mn _{n n} i

i

E e R m d

F f R m d

G g R m d

q q q q q q

e p q q q

e p q q q

e p q q q

-= = = = = =

å ò

å ò

å ò

(22.a)

(

)

(

)

(

)

(

)

(

)

(

)

1 1 1 1 1 1

2 , sin

2 , cos

2 , sin

i i i i i i I j j

mn _n n _i

i I

j j

mn n n i

i I

j j

mn n n i

i

E e R m d

F f R m d

G g R m d

q q q q q q

e p q q q

e p q q q

e p q q q

-= = = = = =

å ò

å ò

å ò

(22.b)

and where j =1, 2, 3, 4, 5 and 6, I is the number of segments, Ri is the coordinate r at the inner

boundary, R_i is the coordinate rat the outer boundary and N is the number of truncation of

the Fourier series. For the nontrivial solution of the system of equations given in Eqs. (19) and (21), the determinant of the coefficient matrix must vanish and these determinants give the fre-quencies of symmetric and antisymmetric modes of vibrations respectively.

4.1 Elliptic cross-sectional plate

The geometry of elliptic cross-sectional ring shaped plate is shown in Figure 1.The geometrical relations of an elliptic ring shaped plate given by Nagaya (1981b) are used for numerical calcula-tion and are given below:

(

)

(

)

(

)

(

)

1 2 2 2 2

1 2 ₁ 2 2

2

1 * *

2 2

*

2

1 * *

2 2

cos sin

2 tan tan , for 2

2, 2

2 tan tan , for 2

i

i i i

i i

i i i

R b a b a b

b a

b a

q q

g p q q p

g p q p

g p q q p

-é ù

= ê + ú

ê ú

ë û

é ù

= - ê ú <

ê ú

ë û

= =

é ù

= + ê ú >

ê ú

ë û

(23.a)

for the outer surface and



₍

₎

₍

₎

(

)

(

)

1 2 2 2 2

1 1 ₁ 1 1

2

1 * *

1 1

*

2

1 * *

1 1

cos sin

2 tan tan , for 2 2, 2

2 tan tan , for 2

i

i i i

i i

i i i

R b a b a b

b a

b a

q q

g p q q p

g p q p

g p q q p

-é ù

= ê_ê + ú_ú

ë û

é ù

= - ê ú <

ê ú

ë û

= =

é ù

= + ê ú >

ê ú

ë û

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

for the inner surface, where a₁ and a₂ are the length of inner and outer semi major axis, and b₁

and b₂ are the length of semi minor axis of an elliptic cross-section. Also q_l* =

(

q_l +q_l-1

)

2 and

i

R is the coordinate r at the i-th boundary, g_i is the angle between the reference axis and the

normal to the segment. For in-plane vibration problem, there exist two types of vibrations; one of which is generated mainly by flexural motion and the other by longitudinal motion.

In the present problem, there are three kinds of basic independent modes of wave propagation have been considered, namely, the longitudinal and two flexural (symmetric and antisymmetric) modes.

5 NUMERICAL ANALYSIS

The numerical analysis of the frequency equation is carried out for heat conducting thermo elastic elliptic cross-sectional plates. The material properties of copper at 42 K are taken approximately as Poisson ratio n =0.3, density r =8.96x103 kg/m3, the Young’s modulus E =2.139x1011 N/m2,

l =8.20x1011 kg/m*s2, m =4.20x1010 kg/m*s2, c_n =9.1x10-2 m2/K*s2, and K =113x10-2 kg*m/K*s2.

The thermal parameters such _a'_, 1

e , e₂, and e₃ are chosen by the following arguments are given by Erbay and Suhubi (1986).

In the numerical calculation, the angle qis taken as an independent variable and the coordi-nate R_i and Ri are at the i-thsegment of the boundary is expressed in terms of q.

Substitut-ing Ri, Ri and the angle gi, between the reference axis and the normal to the i-thboundary

line, the integrations of the Fourier coefficients i n

e , f_ni, g_ni , ein, i n

f , and gi_n can be expressed in

terms of the angle q. Using these coefficients in to equations (20) and (22), the frequencies are obtained for heat conducting thermo elastic elliptical cross-sectional plate.

5.1 Longitudinal mode

The geometrical relations for the elliptic cross-sections given in Eq. (23) are used directly for the numerical calculations, and three kinds of basic independent modes of wave propagation are stud-ied. In case of the longitudinal mode of elliptical cross-section, the cross-section vibrates along the axis of the cylinder, so that the vibration and displacements in the cross-section is symmet-rical about both major and minor axes. Hence, the frequency equation is obtained by choosing both terms of n and m as 0,2, 4, 6,... in Eq. (19) for the numerical calculations. Since the bound-ary of the elliptical cross-sections is irregular in shape, it is difficult to satisfy the boundbound-ary condi-tions along the curved surface, and hence Fourier expansion collocation method is applied. In this method, the curved surface, in the range q =0 and q= p is divided into 20 segments, such that the distance between any two segments is negligible and the integrations is performed for each segment numerically by using the Gauss five point formula .The non-dimensional frequencies are computed for 0< W £1.2, using the secant method.

5.2 Flexural mode

(symmet-Latin American Journal of Solids and Structures 11 (2014) 2589-2606

ric and antisymmetric) modes are considered. The computed non-dimensional frequencies are presented in the form of dispersion curves.

5.3 Quality factor

The ratio of the total elastic energy and the energy loss in one cycle of a material is defined as the quality factor. In engineering and physics, the quality factor of a material is a dimensionless parameter that compares the time constant for decay of an oscillating physical structure’s ampli-tude to its oscillation period. Equivalently, it compares the frequency at which a structure oscil-lates to the rate at which it dissipates its energy. The quality factor is defined as

1 2

F Q

VQ

w

=

where V and Q represents the phase velocity and attenuation coefficient, respectively.

5.4 Dispersion curve

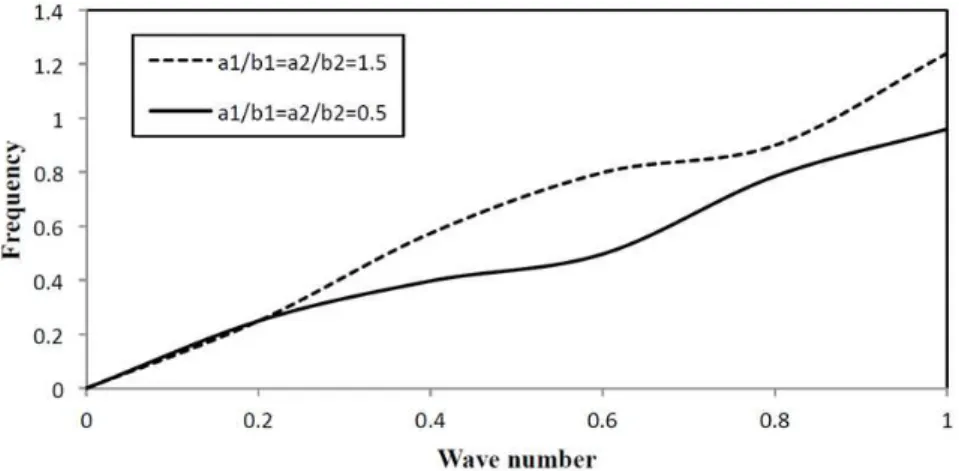
The variation of non-dimensional frequency versus dimensinonless wave number of longitudinal modes of thermo-elastic circular cross sectional plate with and without thermal field for the two values of aspect ratio a b_{1 1} =a b_{2 2} =0.5,1.5 is shown in Figures 2 and 3 respectively. From

Fig-ure 2, it is observed that the dispersion linearly increase with respect to the wave number. For without thermal field, the relation between the wave number and frequency in both the cases of aspect ratios are alike, but for the thermal inclusion the behavior is oscillating throughout the full range of wave number. Figures 4 and 5 shows the dispersion characteristic of flexural (symmetric) modes of elliptic cross-sectional plate with and without thermal field. It shows that, the disper-sion is common for both the plate with and without thermal includisper-sion, where the higher aspect ratio attains higher frequency compared with lower aspect ratio.

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

Figure 3: Non-dimensional wave number versus dimensinonless frequency of longitudinal modes of thermo-elastic circular cross sectional plate.

Figure 4: Non-dimensional wave number versus dimensinonless frequency of flexural symmetric modes of elliptical cross sectional plate.

Latin American Journal of Solids and Structures 11 (2014) 2589-2606 Figure 6: Non-dimensional wave number versus dimensinonless frequency of flexural anti symmetric modes of

elliptical cross sectional plate.

Figure 7: Non-dimensional wave number versus dimensinonless frequency of flexural anti symmetric modes of thermo-elastic elliptical cross sectional plate.

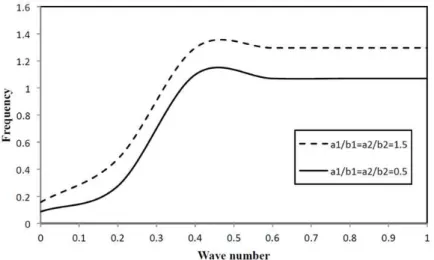
A graph is drawn between non-dimensional wave number versus dimensionless frequency of flex-ural (antisymmetric) modes of elliptic cross-sectional plate with and without thermal environment are shown in Figures 6 and 7 respectively. In both figures, it is observed that as the wave number increases, the non-dimensional frequencies also increases, also it could be inferred that, the disper-sion characteristic are prominent only in lower orders with negligible variations in higher rang. From the figures, it is observed that the non-dimensionless frequency increases with respect to its wave number. It is also observed that, the cross over points between the modes of different aspect ratios of longitudinal and flexural symmetric and antisymmetric modes represents the transporta-tion of energy between the two different vibratransporta-tion medium.

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

respectively. From the Figures 8 and 9, it is observed that, for the increasing values of the aspect ratio modes are merges and oscilating through entire range of frequency. The merging of thermal modes and oscillation of point between the vibrational modes shows that, there is an energy transportation between the modes of vibrations by the effect of aspect ratio and anisotropic of the material.

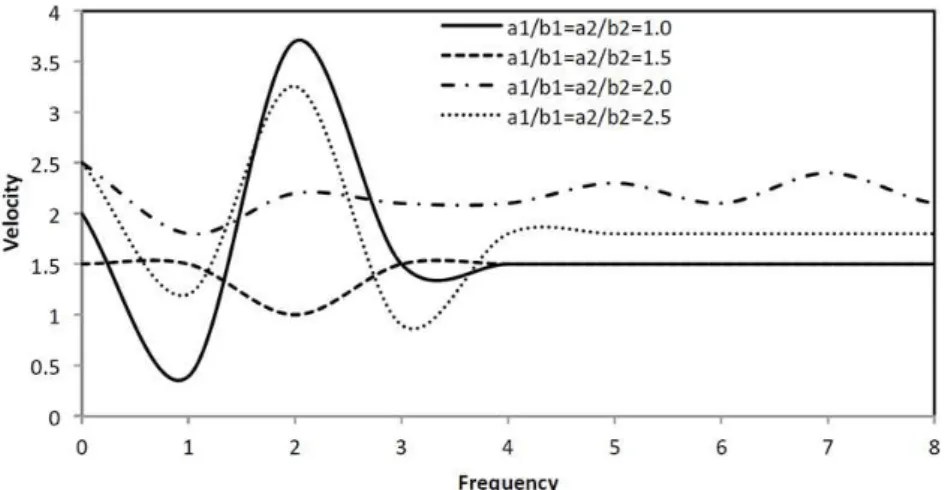
Figures 10 and 11 reveals that the variation of quality factor with the dimensionless frequency for the flexural symmetric modes with and without thermal signal. The quality factor is quite high at lower range of frequency and starts to decay with increasing frequency. The quality factor profile are dispersive in trend for flexural symmetrical modes with thermal signal than in flexural symmetrical modes without thermal signal and experience oscillation in the wave number range

0.2£| |V £0.6 for flexural symmetric thermal modes of vibration. The cross over points between

the vibration modes represents the transfer of energy between the thermal modes.

Figure 8: Variation of velocity versus dimensinonless frequency of flexural symmetric modes of elliptical cross sectional plate.

Latin American Journal of Solids and Structures 11 (2014) 2589-2606 Figure 10: Variation of quality factor versus dimensinonless frequency of flexural symmetric modes of elliptical

cross sectional plate.

Figure 11: Variation of quality factor versus dimensinonless frequency of flexural symmetric modes of thermo-elastic elliptical cross sectional plate.

6 CONCLUSIONS

In this paper, the wave propagation in a heat conducting thermo elastic plate of elliptical shaped is analyzed by satisfying the boundary conditions on the irregular boundary using the Fourier expansion collocation method and the frequency equation for the longitudinal and flexural (sym-metric and antisym(sym-metric) modes of vibrations are obtained. The computed dimensionless fre-quency, velocity and quality factor are plotted in graphs for longitudinal and flexural (symmetric and antisymmetric) modes with and without thermal signals. From the graphical representation, the effect of thermal energy with thermal relaxation times and the anisotropy of the material on the various considered wave characteristics are more significant and dominant in the flexural modes of elliptical cross sectional plate.

References

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

Erbay, E. S., Suhubi, E., S., (1986). Longitudinal wave propagation thermo elastic cylinder. J. Thermal Stresses 9: 279-295.

Green, A. E., Laws, N., (1972). On the Entropy Production Inequality. Arch. Rational Mech. Anal., 45:47-53. Green, A. E., Lindsay, K.A., (1972). Thermoelasticity. J. Elasticity 2: 1-7.

Gaikwad, M.K., Desmukh, K.C., (2005). Thermal deflection of an inverse thermo elastic problem in a thin iso-tropic circular plate. J. Applied Mathematical modeling 29: 797-804

Heyliger, P.R., Ramirez, G., (2000). Free vibration of Laminated circular piezoelectric plates and disc. J. Sound and Vib., 229(4): 935-956.

Kumar, R., Partap, G., (2007). Free vibration of microstretch thermo elastic plate with one relaxation time. J. theoretical and applied fracture mechanics. 48: 238-257.

Lord, H. W., Shulman, Y., (1967). A generalized dynamical theory of thermo elasticity. J. Mech Phys Solids., 5: 299-309.

Mirsky, I., (1964). Wave propagation in transversely isotropic circular cylinders. J. of Acoust. Soc. of Am. 36: 41-51.

Nagaya, K., (1981a). Simplified method for solving problems of plates of doubly connected arbitrary shape, Part I: Derivation of the frequency equation. Journal of Sound and Vibration74(4): 543-551.

Nagaya, K., (1981b). Simplified method for solving problems of plates of doubly connected arbitrary shape, Part II: Applications and experiments. Journal of Sound and Vibration 74(4): 553-564.

Nagaya, K., (1983a). Vibration of a thick walled pipe or ring of arbitrary shape in its plane. Journal of Applied Mechanics, Vol.50: 757-764.

Nagaya, K., (1983b). Vibration of a thick polygonal ring in its plane. Journal of Acoustical Society of America 74(5): 1441-1447.

Nagaya, K., (1983c). Direct method on the determination of eigen frequencies of arbitrary shaped plates. Journal of App. Mech. 105: 132-136.

Selvamani, R., Ponnusamy, P., (2012). Damping of generalized thermoelastic waves in a homogeneous isotropic plate. Mater .Phys .Mechs. 14: 64-73.

Sharma, J.N., Pathania, V, P., (2005). Generalized thermo elastic wave propagation in circumferential direction of transversely isotropic cylindrical curved plates. J. Sound and Vib. 2 (74): 747-759.

Ponnusamy, P., Selvamani, R., (2012). Dispersion analysis of generalized magneto-thermo elastic waves in a transversely isotropic cylindrical panel. J.Therm Stresses 35(12): 1119-1142.

Sharma, J.N., Kumar, R., (2004). Asymptotic of wave motion in transversely isotropic plates. J. Sound and Vib. 274: 747-759.

Tso, Y.K., Hansen, C.H., (1995). Wave propagation through cylinder/plate junctions. J. Sound and Vib. 186(3): 447-461.

Verma, K.L., Hasebe, N., (2001). Wave propagation in plates of general anisotropic media in generalized thermoe-lasticity. Int. J. Eng. Sci. 39: 1739-1763.

Latin American Journal of Solids and Structures 11 (2014) 2589-2606 Appendix A

The expressions i i n n

e k used in Eqs. (20) and (22) are given as follows:

{

}

(

)

{

}

(

)

{

}

1

2 2 2

3

1

2 ( 1) ( ) ( ) ( ) cos 2( )cos

( ) 2 cos ( ) 1 cos

2 ( 1) ( ) ( ) ( ) sin sin 2( ), 1, 2

i

n n i i n i i

i i i n i

n i i n i i

e n n J ax ax J ax n

x a d i J ax n

n n J ax ax J ax n i

a a a q g q

a l q g l e a q

a a a q q g

+ +

= - +

-é ù

- +_êë + - _úû+ + W

+ - - - =

(A.1)

{

}

{

}

3

3 3 1 3

2

3 3 3 1 3

2 ( 1) ( ) ( ) ( ) cos cos 2( )

2 [ ( 1) ( ) ] ( ) ( ) ( ) sin sin 2( )

n n n i

n n i

e n n J ax ax J ax n

n n ax J ax ax J ax n

a a a q q g

a a a a q q g

+

+

= - -

-+ - - + - (A.2)

{

}

{

}

4

3 3 1 3

2

3 3 3 1 3

2 ( 1) ( ) ( ) ( ) cos cos 2( )

2 [ ( 1) ( ) ] ( ) ( ) ( ) sin sin 2( )

n n n i

n n i

e n n Y ax ax Y ax n

n n ax Y ax ax Y ax n

a a a q q g

a a a a q q g

+

+

= - -

-+ - - + - (A.3)

{

}

(

)

{

}

(

)

{

}

1

2 2 2

3

1

2 ( 1) ( ) ( ) ( ) cos 2( ) cos

( ) 2 cos ( ) 1 cos

2 ( 1) ( ) ( ) ( ) sin sin 2( ), 5, 6

i

n n i i n i i

i i i n i

n i i n i i

e n n Y ax ax Y ax n

x a d i Y ax n

n n Y ax ax Y ax n i

a a a q g q

a l q g l e a q

a a a q q g

+ +

= - +

-é ù

- +_êë + - _úû+ + W

+ - - - = (A.4)

{

}

{

}

2 1 1

2 [ ( 1) ( ) ] ( ) ( ) ( ) cos sin 2( ) 2 ( ) ( ) ( 1) ( ) sin cos 2( ), 1, 2

i

n i n i i n i i

i n i n i i

f n n ax J ax ax J ax n

n ax J ax n J ax n i

a a a a q q g

a a a q q g

+ +

= - - +

-+ - - - = (A.5)

{

}

{

}

3

3 3 1 3

2

3 1 3 3 3

2 ( 1) ( ) ( ) ( ) cos sin 2( )

2( ) ( ) [( ) 2 ( 1)] ( ) sin cos 2( )

n n n i

n n i

f n n J ax ax J ax n

ax J ax ax n n J ax n

a a a q q g

a a a a q q g

+ +

= - -

-- - - (A.6)

{

}

{

}

4

3 3 1 3

2

3 1 3 3 3

2 ( 1) ( ) ( ) ( ) cos sin 2( )

2( ) ( ) [( ) 2 ( 1)] ( ) sin cos 2( )

n n n i

n n i

f n n Y ax ax Y ax n

ax Y ax ax n n Y ax n

a a a q q g

a a a a q q g

+ +

= - -

-- - - (A.7)

{

}

{

}

2

1

1

2 [ ( 1) ( ) ] ( ) ( ) ( ) cos sin 2( ) 2 ( ) ( ) ( 1) ( ) sin cos 2( ), 5, 6

i

n i n i i n i i

i n i n i i

f n n ax Y ax ax Y ax n

n ax Y ax n Y ax n i

a a a a q q g

a a a q q g

+ +

= - - +

-+ - - - = (A.8)

(

)

(

) (

)

(

)

{

cos 1 1 cos( )cos

}

, 1,2

i

n i i n i i n i i

k =d n n- q+g J aax - aax J ₊ aax q-g nq i = (A.9)

3 _0.0

n

k = , k_n4 =0.0 (A.10)

(

)

(

) (

)

(

)

{

cos 1 1 cos( )cos

}

, 5, 6

i

n i i n i i n i i

k =d n n- q+g Y aax - aax Y ₊ aax q-g nq i = (A.11)

{

}

(

)

{

}

(

)

{

}

1

2 2 2

3

1

2 ( 1) ( ) ( ) ( ) cos 2( )sin

( ) 2 cos ( ) 1 cos

2 ( 1) ( ) ( ) ( ) cos sin 2( ), 1,2

j

n _{n i i n i i}

i i i n i

n i i n i i

e n n J ax ax J ax n

x a d i J ax n

n n J ax ax J ax n i

a a a q g q

a l q g e a q

a a a q q g

+ +

= - +

-é ù

- +_êë + - _úû+ + W

- - - - =

Latin American Journal of Solids and Structures 11 (2014) 2589-2606

{

}

{

}

3

3 3 1 3

2

3 3 1 3

2 ( 1) ( ) ( ) ( ) sin cos 2( )

2 [ ( 1) ( ) ] ( ) ( ) ( ) cos sin 2( )

n _{n n i}

i n n i

e n n J ax ax J ax n

n n ax J ax ax J ax n

a a a q q g

a a a a q q g

+

+

= - -

-- - - + - (A.13)

{

}

{

}

4

3 3 1 3

2

3 3 1 3

2 ( 1) ( ) ( ) ( ) sin cos 2( )

2 [ ( 1) ( ) ] ( ) ( ) ( ) cos sin 2( )

n _{n n i}

i n n i

e n n Y ax ax Y ax n

n n ax Y ax ax Y ax n

a a a q q g

a a a a q q g

+

+

= - -

-- - - + - (A.14)

{

}

(

)

{

}

(

)

{

}

1

2 2 2

3

1

2 ( 1) ( ) ( ) ( ) cos 2( )sin

( ) 2 cos ( ) 1 cos

2 ( 1) ( ) ( ) ( ) cos sin 2( ), 5, 6

j

n _{n i i n i i}

i i i n i

n i i n i i

e n n Y ax ax Y ax n

x a d i Y ax n

n n Y ax ax Y ax n i

a a a q g q

a l q g e a q

a a a q q g

+ +

= - +

-é ù

- +_êë + - _úû+ + W

- - - - =

(A.15)

{

}

{

}

2

1

1

2 [ ( 1) ( ) ] ( ) ( ) ( ) sin sin 2( ) 2 ( ) ( ) ( 1) ( ) cos cos 2( ), 1, 2

i

n i n i i n i i

i n i n i i

f n n ax J ax ax J ax n

n ax J ax n J ax n i

a a a a q q g

a a a q q g

+ +

= - - +

-- - - - = (A.16)

{

}

{

}

3

3 3 1 3

2

3 1 3 3 3

2 ( 1) ( ) ( ) ( ) sin sin 2( )

2( ) ( ) [( ) 2 ( 1)] ( ) cos cos 2( )

n n n i

n n i

f n n J ax ax J ax n

ax J ax ax n n J ax n

a a a q q g

a a a a q q g

+ +

= - -

-+ - - - - (A.17)

{

}

{

}

4

3 3 1 3

2

3 1 3 3 3

2 ( 1) ( ) ( ) ( ) sin sin 2( )

2( ) ( ) [( ) 2 ( 1)] ( ) cos cos 2( )

n n n i

n n i

f n n Y ax ax Y ax n

ax Y ax ax n n Y ax n

a a a q q g

a a a a q q g

+ +

= - -

-+ - - - - (A.18)

(

)

(

) (

)

(

)

{

cos 1 1 cos( )sin

}

, 1,2

i

n _{i i n i i n i i}

k =d n n- q+g J aax + aax J ₊ aax q-g nq i= (A.19)

3

0.0

n

k = , kn4 =0.0 (A.20)

(

)

(

) (

)

(

)

{

cos 1 1 cos( )sin

}

, 5, 6

i

n _{i i n i i n i i}