Vortex Lattie of a Long Superonduting
Wire with a Columnar Defet
Edson Sardellaand Rodrigo de AlvarengaFreire
Departamentode Fsia,FauldadedeCi^enias
UniversidadeEstadual Paulista
CaixaPostal473,17033-360, Bauru-SP,Brazil
Reeivedon28February,2002
Inthepresentworkwe studyalongsuperondutingwirewithaolumnardefetinthepresene
ofanappliedmagnetield. Therosssetion oftheylinderisassumedtobeirular. Theeld
is taken uniformand parallel to theylinder axis. We use theLondon theoryto investigate the
vortexlattieinsidethewire. Althoughthistheoryisvalidinthelimitoflowvortexdensity,that
is, when the nearest neighbor vortex distane is muh larger than the oherene length, we an
obtainareasonablequalitativedesriptionoflattieproperties. Wealulate: (1)thevortexlattie
strutureusingthesimulatedannealingtehnique;(2)themagnetizationurveasafuntionofthe
appliedeld.
I Introdution
Reentprogressesin nanotehnologyhavemade
possi-ble thefabriationof mesosopisuperonduting
ma-terialswiththesizeomparabletotheoherenelength
() and London penetration depth (). This has
mo-tivatedbothexperimentalandtheoretialphysiiststo
investigate the magneti properties of these small
su-perondutors. The properties of the vortex lattie,
forinstane,insuperondutorsofonnedgeometries
hange radially with respet to the behavior in the
bulk.
Previousworkshavebeendediated tothe
investi-gationofasuperondutingwire[1,2,3℄. Inthispaper
weextendthesestudiestotheaseofasuperonduting
wirewithaolumnardefet.
II The loal magneti eld
The geometry of the problem we will onsider in this
workisillustratedinFig.1. Theappliedmagnetield
H isparallelto theylinderaxisandisdenotedbyH.
The internal and externalradius of theylinder are a
andb respetively.
&% '$ q q q q q q q
q q q q q q q q
q
q
q
q
q
q
q
q
q
q q
q q
q q
q q b
a H
Figure 1. Cross setion of a long superonduting
ylin-derwithaolumnardefet. Thedotsrepresenttheapplied
magnetieldwhihisdiretedalongtheylinderaxis.
ThestartingpointofourstudyistheLondon
equa-tionfortheloalmagnetieldofaverystrongtype-II
superondutorforwhihtheGinzburg-Landau
param-eter =
1. For our purposes, this equation an
bemoreonvenientlywritteninylindrialoordinates
as
2
2
h
2
+ 1
h
+
1
2
2
h
' 2
+h=
0 N
X
i=1
Æ(
i );
(1)
where
i
istheposition ofthei-vortexline.
This isa Dirihlet problem in whih theboundary
onditionsaregivenby
h(a;')=h(b;')=H : (2)
The solution of the London equation under these
boundary onditions may be found by means of the
h(;')=
0 N
X
i=1
G(;';
i ;'
i )+h
1
(): (3)
Thesolutionontainstwodistintparts[4℄. Therst
termisapartiularsolutionoftheLondonequationas
ifnoappliedeldwerepresent. Theseondtermisthe
solution of the homogeneous London equation in the
abseneofanyvortexline(Meissnerstate).
TheGreen'sfuntion G((;'; 0
;' 0
)hastheform
G(;';0;'0)= 1
2 1
X
m= 1 e
im(' ' 0
)
g
m (;
0
); (4)
wheretheFourieroeÆientsaregivenby
g
m (;
0
)= (
m (
0
;b)
m (a;)
2
m(a;b)
; a
0
m( 0
;a)m(b;)
2
m (a;b)
;
0
b; (5)
where
m
(x;y)=K
m (x=)I
m
(y=) K
m (y=)I
m (x=):
(6)
Here K
m
(x) and I
m
(x) are theBessel funtions of
seondkind.
The homogeneoussolution an be easily found by
usingstandardmethodsofsolutionofdierential
equa-tions. Onehas
h
1
()=H
0
(a;)
0 (b;)
0 (a;b)
: (7)
III The free energy
Wewillmoveforwardonthedeterminationofthe
Lon-donfreeenergy(theHelmholtzfreeenergyinthe
ther-modynami ontext). In the London approximation
thisenergy(perunit volume)isgivenby
F= 1
8A Z
2
0 Z
b
a "
2
2
h
'
2
+ 2
h
2
+h 2
#
dd'; (8)
d
whereA=(b 2
a 2
)istheareaoftherosssetionof
theylindrialshell.
TheLondonfreeenergyFisathermodynami
fun-tionofthemagnetiindutionB,whihisinonvenient
foralulationsinvolvingaxedappliedmagnetield.
Therefore,itisneessarytouseaLegendre
transforma-tionfortheGibbs freeenergy
G=F BH
4
; (9)
where B isthe magnetiindution whih isdened as
aspatialaverageof theloal magnetield
B = 1
A Z
hd 2
: (10)
Bymeans of these denitions of F and B, after a
tediousbutstraightforwardalulation,wendforthe
Gibbs freeenergy
G =
2
0
8A N
X
i;j=1 G(
i ;'
i ;
j ;'
j )+
0 H
4A N
X
i=1
0 (a;
i
)
0 (b;
i )
0 (a;b)
N
0 H
4A
2
H 2
4A (a;b)
b
Æ
0 (a;b)+
a
Æ
0
(b;a) 2
where weusedthfollowingdenition
Æ
m
(x;y)=K
m (x)I
m+1
(y)+K
m+1 (y)I
m
(x): (12)
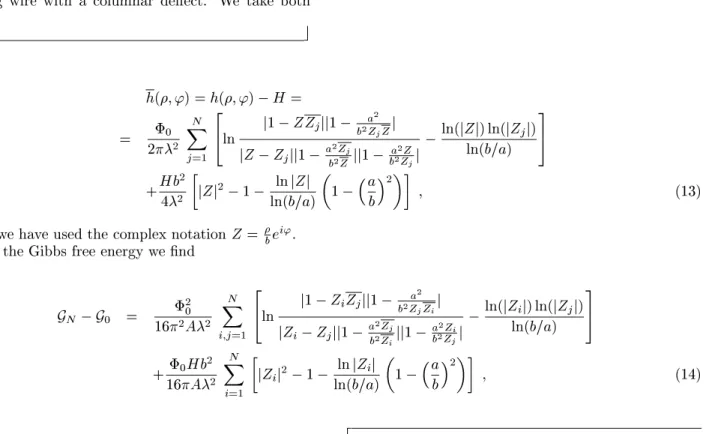
IV The limit of a thin wire
We will now take the limit of a very thin
superon-duting wire with a olumnar deet. We take both
aand b. Thefollowingresultsarealso valid
for both a small dis (if a = 0) and a small ring (if
a 6= 0)[3℄. Within this limit the loal magneti eld
and the Gibbs free energy are enormously simplied.
Fortheloalmagnetield weobtain
h(;')=h(;') H=
=
0
2 2
N
X
j=1 2
4
ln
j1 ZZ
j jj1
a 2
b 2
ZjZ j
jZ Z
j jj1
a 2
Zj
b 2
Z jj1
a 2
Z
b 2
Z
j j
ln(jZj)ln(jZ
j j)
ln(b=a) 3
5
+ Hb
2
4 2
jZj 2
1
lnjZj
ln(b=a)
1
a
b
2
; (13)
where wehaveusedtheomplexnotationZ =
b e
i'
.
FortheGibbs freeenergywend
G
N G
0 =
2
0
16 2
A 2
N
X
i;j=1 2
4
ln
j1 Z
i Z
j jj1
a 2
b 2
ZjZi j
jZ
i Z
j jj1
a 2
Z
j
b 2
Zi jj1
a 2
Zi
b 2
Z
j j
ln(jZ
i j)ln(jZ
j j)
ln(b=a) 3
5
+
0 Hb
2
16A 2
N
X
i=1
jZ
i j
2
1
lnjZ
i j
ln(b=a)
1
a
b
2
; (14)
d
whereG
0
istheGibbsfreeenergyintheMeissnerstate.
These resultsarein agreementwiththose of[3℄in the
partiularasea=0.
V Results and Disussion
Inthemixed statethemagnetization showsaseriesof
piksasafuntionof theappliedmagnetield. Eah
pikisgenerallyinterpretedasanindiationofthe
en-traneofanindividualvortexinthesample. Soonafter
the entraneof avortex, theyrearrangethemselvesin
ordertolowertheGibbsfreeenergy. Thisonguration
orrespondsto theglobal optimum. Eah entraneof
avortexisassoiatedwitharitialeld,thesoalled
mathing eld. These eldswere foundas follows. As
wehavestatedpreviously,thevortiespenetrateoneat
thetime. AtthetransitionfromNtoN+1vorties,we
assume that theGibbs free energy isontinuous, that
is, G
N
(H)= G
N+1
(H). The solutionof this equation
providesthevalueofthemathingeld. Fulldetailsof
howtodeterminethesemathingeldsanbefoundin
Ref. [2℄.
ThemathingeldsweredetermineduptoN =24
zation4M=B H. Theresultisdepitedin Fig.2.
0.0
0.2
0.4
0.6
0.8
-1.4x10
-4
-1.2x10
-4
-1.0x10
-4
-8.0x10
-5
-6.0x10
-5
-4.0x10
-5
-2.0x10
-5
0.0
4
π
M/H
c2
H/H
c2
Figure2.Magnetizationversustheappliedeldfor=100,
a=,andb=10.Theeldsareinunitsoftheupper
rit-ialeldH2.
As an be seen in this gure, the magnetization
pene-remainsnegativeforallvaluesof H until N =24.
Re-ently, someauthors[3, 5℄ suggestedthat foronned
geometriesofdimensionsomparabletothe
fundamen-tallengths(;),themagnetizationpresentsapositive
sign. This indiatesthat thevortexsystemmaybe in
a paramagneti Meissner state. This has been
inter-pretedasamanifestationofeitherametastability[3,5℄
ofthevortexsystemortheformationofagiantvortex
state[6℄. Withinourapproah,wewillneverbeableto
detettheformationthesevortexstates. First,we
an-notndgiantvortexstatesduetoalimitationof
Lon-don theory whih supposes that the nearest neighbor
distanesbetweenthevortiesis muh largerthanthe
oherenelength. Seond,weannotnd
metastabil-itysineweusethesimulatedannealingmethodwhih
rarelystopsat aloalminimumofthevortexstate.
-1.00
-0.75
-0.50
-0.250.00 0.25 0.50 0.75 1.00
-1.00
-0.75
-0.50
-0.25
0.00
0.25
0.50
0.75
1.00
Figure3. Distributionoftheloalmagnetieldh (;')for
N =10vorties. Thelengthsare inunitsofb. Thedarker
regionsindiatewheretheeldismoreintense.
We also determined the distribution of the loal
magneti eld for twovalues of N. As we ansee in
Fig.3,thevortiesaredistributedinaringaroundthe
olumnardeet. However,forN =11this singlering
regime of asingle-to amulti-ring vortex stateanbe
learlyseenin Fig.2informof apikat H=0:45H
2
.
-1.00
-0.75
-0.50
-0.250.00 0.25 0.50 0.75 1.00
-1.00
-0.75
-0.50
-0.25
0.00
0.25
0.50
0.75
1.00
Figure4. ThesameasFig.3,exeptthatnowN=11.
Anowledgement
WethanktheS~aoPauloAgenyFAPESPfor
nan-ialsupport.
Referenes
[1℄ G.Bobel,NuovoCimento38,6320(1965).
[2℄ P.A.VenegaseEdsonSardella,Phys.Rev.B58,5789
(1998).
[3℄ E.Akkermans,D.M.Gangart,eK.Mallik,Phy.Rev.
B63,4523(2001).
[4℄ Detailed solution of the London equation for the
presentgeometrywillbegivenelsewhere.
[5℄ A. K.Geim, S.V. Dubonos,J.G.S.Lok,M. Henini,
eJ.C.Maan,Nature,396,144(1998).